Numerical Simulation of Nonlinear and Non-Isothermal Liquid Chromatography for Studying Thermal Variations in Columns Packed with Core-Shell Particles

https://doi.org/10.46481/jnsps.2023.1350

Authors

  • Abdulaziz G. Ahmad Department of Mathematics Programme, National Mathematical Centre Abuja, Nigeria; Department of Applied Mathematics, Federal University of Technology Babura, Nigeria
  • Nnamdi F. Okechi Department of Mathematics Programme, National Mathematical Centre Abuja, Nigeria
  • David U. Uche Department of Mathematics Programme, National Mathematical Centre Abuja, Nigeria; Department of Mathematics, University of Abuja, Nigeria
  • Abdulwasiu O. Salaudeen Department of Applied Mathematics (Chemistry Unit) Programme, National Mathematical Centre Abuja, Nigeria

Keywords:

Non-isothermal chromatography, Non-linear isotherm, One-dimensional lumped kinetic model, High-resolution scheme

Abstract

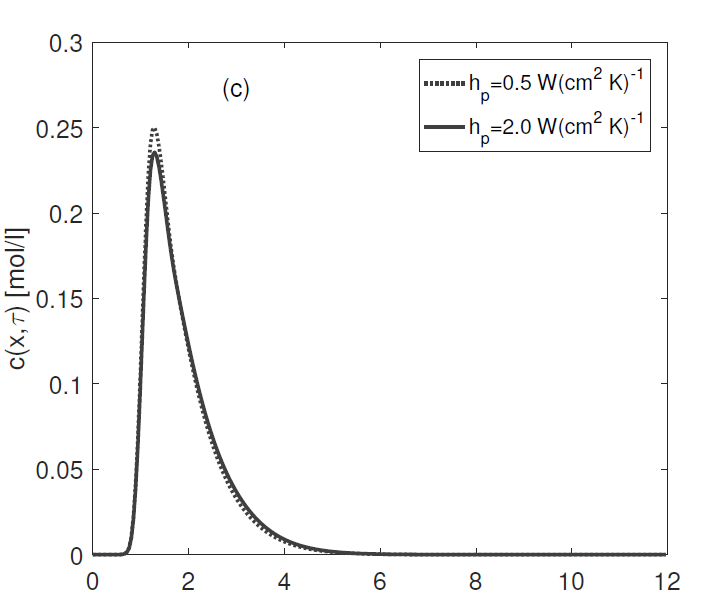
A high-resolution flux-limiting semi-discrete finite volume scheme (HR-FVS) is applied in this study to numerically approximate the nonlinear and non-isothermal flow of one-dimensional lumped kinetic model (1D-LKM), for a fixed-bed column loaded with core-shell particles. The developed model comprise a system of convection-dominated partial differential for mass and energy balances in the mobile phases coupled with differential equation and algebraic equation in the stationary phase. The solution of the model equations is obtained by utilizing a HR-FVS, the scheme has second-order accuracy even on the grid coarse and its explicit nature has the potential to resolve the arisen sharp discontinuities in the solution profiles. A second-order total variation diminishing (TVD) Runge-Kutta technique is used to solve the system of ODEs in time. Several forms of a single-solute mixture are produced to investigate the influences of the fractions of core radius on thermal waves and concentration fronts. Moreover, a particular criterion is introduced for analyzing the performance of the underlying process and to identify the optimal parameter values of the fraction of core radius.

Dimensions

G. Guiochon, A. Felinger, D. G. Shirazi & A. M. Katti, Fundamentals of Preparative and Nonlinear Chromatography, Elsevier Academic Press, New York (2006). DOI: https://doi.org/10.1016/B978-012370537-2/50030-8

D. M. Ruthven, Principles of Adsorption and Adsorption Processes, Wiley-Interscience, New York (1984).

G. Guiochon, “Preparative Liquid Chromatography”, Journal of Chromatography A 965 (2002) 129. DOI: https://doi.org/10.1016/S0021-9673(01)01471-6

T. Gu, M. Liu, K. S. C. Cheng, S. Ramaswamy, & C. A. Wang, “General rate model approach for the optimization of the core radius fraction for multicomponent isocratic elution in preparative nonlinear liquid chromatography using cored beads”, Chemical Engineering Science 66 (2011) 3531. DOI: https://doi.org/10.1016/j.ces.2011.04.021

J. J. Kirkland, F. A. Truszkowski, C. H. Dilks & G. S. Engel, “Superficially porous silica microspheres for fast high-performance liquid chromatography of macromolecules”, Journal of Chromatography A 890 (2000) 3. DOI: https://doi.org/10.1016/S0021-9673(00)00392-7

Y. Q. Xiang, B. W. Yan, C. V. McNe , P. W. Carr & M. L. Lee, “Synthesis of micron diameter polybutadiene-encapsulatednon-porous zirconia particles forultra-high pressure liquid chromatography”, Journal of Chromatography A 983 (2003) 83.

J. Ning, F. Z. Kong, D. H. Li & Y. Z. Du, “Preparation of monodisperse agglomerated pellicular anion-exchange resins compatible with highperformance liquid chromatography solvents for ion chromatography”, Journal of Chromatography A 793 (1998) 193. DOI: https://doi.org/10.1016/S0021-9673(97)00889-3

K. Kaczmarski & G. Guiochon, “Modeling of the mass-transfer kinetics in chromatographic columns packed with shell and pellicular particles”, Analytical Chemistry 79 (2007) 4648. DOI: https://doi.org/10.1021/ac070209w

U. D. Uche, M. Uche, & F. Okafor, “Numerical solution of a two-dimensional model for non-isothermal chromatographic reactor packed with core-shell particles”, Journal of the Nigerian Mathematical Society 3 (2021) 97.

U. D. Uche, M. Uche, F. Okafor & K. Utalor, “Modeling and simulation of isothermal reactive liquid chromatography for two component elutione effects of core-shell particles”, International Journal of Mathematical Sciences and Optimization: Theory and Applications 8 (2022) 117.

U. D. Uche & M. Uche, “Theoretical Analysis of Linearized Nonisothermal Two-dimensional Model of Liquid Chromatography Columns Packed with Core-Shell Particles”, International Journal of Applied and Computational Mathematics 7 (2021) 83. DOI: https://doi.org/10.1007/s40819-021-01025-2

A. Brandt, G. Mann & W. Arlt, “Temperature Gradients in Preparative High-performance Liquid Chromatography Columns”, Journal of Chromatography A 768 (1997) 109. DOI: https://doi.org/10.1016/S0021-9673(97)00235-5

T. Sainio, M. Kaspereit, A. Kienle & A. Seidel-Morgenstern, “Thermal Effects in Reactive Liquid Chromatography”, Chemical Engineering Science 62 (2007) 5674. DOI: https://doi.org/10.1016/j.ces.2007.02.033

H. Poppe, J. C. Kraak, J. F. K. Huber & J. H. M. Van den Berg, “Temperature Gradients in HPLC Columns Due to Viscous Heat Dissipation”, Chromatographia 14 (1981) 515. DOI: https://doi.org/10.1007/BF02265631

A. G. Ahmad & S. Qamar, “Effect of temperature variations on nonequilibrium and non-isothermal two-component liquid chromatography in cylindrical columns”, Journal of Liquid Chromatography and Related Technology 43 (2020) 890. DOI: https://doi.org/10.1080/10826076.2020.1848863

A. G. Ahmad, S. Qamar & A. Seidel-Morgenstern, “Linearized nonequilibrium and non-isothermal two-dimensional model of liquid chromatography for studying thermal effects in cylindrical columns”, Journal of Liquid Chromatography and Related Technology 42 (2019) 436. DOI: https://doi.org/10.1080/10826076.2019.1625370

H. W. Haynes Jr, “An Analysis of Sorption Heat Effects in the Pulse Gas Chromatography Di usion Experiment”, AIChE Journal 32 (1986) 1750. DOI: https://doi.org/10.1002/aic.690321021

Y. Guillaume & C. Guinchard, “Prediction of Retention Times, Column Efficiency, and Resolution in Isothermal and Temperature-programmed Gas Chromatography: Application for Separation of Four Psoalens”, Journal of chromatography Science 35 (1997) 14. DOI: https://doi.org/10.1093/chromsci/35.1.14

G. Peter, Robinson & L. O. Allan, “Comparison of Isothermal and Nonlinear Temperature Programmed Gas Chromatography the Temperature Dependence of the Retention Indices of a Number of Hydrocarbons on Squalane and SE-30”, Journal of Chromatography A 57 (1971) 11. DOI: https://doi.org/10.1016/0021-9673(71)80002-X

F. Gritti, M. Michel Martin & G. Guiochon, “Influence of Viscous Friction Heating on the Efficiency of Columns Operated Under Very High Pressures”, Analytical Chemistry 81 (2009) 3365. DOI: https://doi.org/10.1021/ac802632x

T. Teutenberg, High-temperature Liquid Chromatography: A Users Guide for Method Development, RSC Chromatography Monographs (2010).

F. D. Antia & C. Horvath, “High-performance Liquid Chromatography at Elevated Temperatures: Examination of Conditions for the Rapid Separation of Large Molecules”, Journal of Chromatography A 435 (1988) 1. DOI: https://doi.org/10.1016/S0021-9673(01)82158-0

D. C. Snyder & J. W. Dolan, “Initial Experiments in High-performance Liquid Chromatographic Method Development I. Use of a Starting Gradient Run”, Journal of Chromatography A 721 (1996) 3. DOI: https://doi.org/10.1016/0021-9673(95)00770-9

L. R. Snyder, J. W. Dolan & D. C. Lommen, “Drylabr Computer Simulation for High-performance Liquid Chromatographic Method Development: I. Isocratic Elution”, Journal Chromatography A 485 (1989) 45. DOI: https://doi.org/10.1016/S0021-9673(01)89133-0

A. I. Anya, U. Ofe & A. Khan, “Mathematical Modeling of Waves in a Porous Micropolar Fibrereinforced Structure and Liquid Interface”, Journal of the Nigerian Society of Physical Sciences 4 (2022) 823. DOI: https://doi.org/10.46481/jnsps.2022.823

F. O. Akinpelu, R. A. Oderinu & A. D. Ohagbue, “Analysis of hydromagnetic double exothermic chemical reactive flow with connective cooling through a porous medium under bimolecular kinetics”, Journal of the Nigerian Society of Physical Sciences 4 (2022) 130. DOI: https://doi.org/10.46481/jnsps.2022.525

M. Suzuki & J. M. Smith, “Kinetic Studies by Chromatography”, Chemical Engineering Science 26 (1971) 221. DOI: https://doi.org/10.1016/0009-2509(71)80006-4

S. Qamar, N. Kiran, T. Anwar, S. Bibi & A. Seidel-Morgenstern, “Theoretical Investigation of Thermal Effects in an Adiabatic Chromatographic Column Using a Lumped Kinetic Model Incorporating Heat Transfer Resistances”, Indusrial & Engineering Chemical Research 57 (2015) 2287. DOI: https://doi.org/10.1021/acs.iecr.7b04555

K. Horv´ath & A. Felinger, “Influence of particle size and shell thickness of core-shell packing materials on optimum experimental conditions in preparative chromatography”, Journal of Chromatography A 407 (2015) 100. DOI: https://doi.org/10.1016/j.chroma.2015.06.037

S. Qamar, S, Perveen & A. Seidel-Morgenstern, “Numerical Approximation of Nonlinear and Non-equilibrium Two-dimensional Model of Chromatography”, Computers & Chemical Engineering 94 (2016) 411. DOI: https://doi.org/10.1016/j.compchemeng.2016.08.008

P. V. Danckwerts, “Continuous flow systems”, Chemical Engineering Science 2 (1953) 1. DOI: https://doi.org/10.1016/0009-2509(53)80001-1

T. D. Vu & A. Seidel-Morgenstern, “Quantifying temperature and flow rate effects on the performance of a fixed-bed chromatographic reactor”, Journal of Chromatography A 1218 (2011) 8097. DOI: https://doi.org/10.1016/j.chroma.2011.09.018

Published

2023-04-24

How to Cite

Ahmad, A. G., Okechi, N. F., Uche, D. U., & Salaudeen, A. O. (2023). Numerical Simulation of Nonlinear and Non-Isothermal Liquid Chromatography for Studying Thermal Variations in Columns Packed with Core-Shell Particles. Journal of the Nigerian Society of Physical Sciences, 5(2), 1350. https://doi.org/10.46481/jnsps.2023.1350

Issue

Volume 5, Issue 2, May 2023 (In Progress)

Section

Original Research
Copyright & Licensing

Copyright (c) 2023 Abdulaziz G. Ahmad, Nnamdi F. Okechi, David U. Uche, Abdulwasiu O. Salaudeen

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The Journal of the Nigerian Society of Physical Sciences (JNSPS) is published under the Creative Commons Attribution 4.0 (CC BY-NC) license. This license was developed to facilitate open access, namely, it allows articles to be freely downloaded and to be re-used and re-distributed without restriction, as long as the original work is correctly cited. More specifically, anyone may copy, distribute or reuse these articles, create extracts, abstracts, and other revised versions, adaptations or derivative works of or from an article, mine the article even for commercial purposes, as long as they credit the author(s).

Crossref
Scopus
Google Scholar
Europe PMC