Thermal instability of rotating Jeffrey nanofluids in porous media with variable gravity

Authors

  • Pushap Lata Sharma Department of Mathematics & Statistics, Himachal Pradesh University, Summer Hill, Shimla, India
  • Deepak Bains Department of Mathematics & Statistics, Himachal Pradesh University, Summer Hill, Shimla, India
  • Pankaj Thakur aculty of Science and Technology, ICFAI University, Baddi, Solan, India https://orcid.org/0000-0001-8119-2697

Keywords:

nanofluid, variable gravity, porous medium, Galerkin method, rotation

Abstract

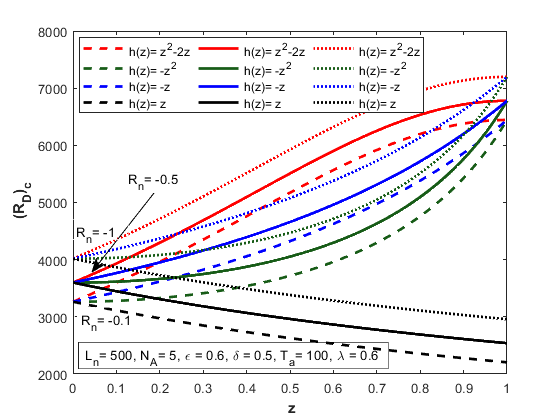
It is investigated how changes in gravity affect the thermal instability rotating Jeffrey nanofluids in porous media. Along with the Galerkin method and normal mode approach, the Darcy model is used. The distinct variable gravity parameters taken in this paper are: h(z)=z2-2z, h(z)=-z2, h(z)=-z and h(z)=z and their effects on the Jeffrey parameter, Taylor number, moderated diffusivity ratio, porosity of porous media, Lewis number and nanoparticle Rayleigh number on stationary convection have been scrutinized and graphically shown. Our finding demonstrates that varying gravity parameter h(z)=z2-2z has more stabilising impact on stationary convection. We have also discovered the necessary condition for overstability in the instance of oscillatory convection for this problem.

Dimensions

S.U. Choi & J. A. Eastman, “Enhancing thermal conductivity of fluids with nanoparticles”, Argonne National Lab.(ANL) (1995).

J. Buongiorno, “Convective transport in nanofluids”, Transactions of the ASME 128 (2006) 240.

S. C. Tzeng, C. W. Lin & K. Huang, “Heat transfer enhancement of nanofluids in rotary blade coupling of four-wheel-drive vehicles”, Acta Mechanica 179 (2005) 11.

S. Kim, I. C. Bang, J. Buongiorno & L. Hu, “Study of pool boiling and critical heat flux enhancement in nanofluids”, Bulletin of the Polish Academy of Sciences: Technical Sciences 55 (2007) 211.

J. Routbort, et al., “Argonne national lab, michellin north america, st.”, Gobain Corp (2009).

G. Donzelli, R. Cerbino & A. Vailati, “Bistable heat transfer in a nanofluid”, Physical review letters 102 (2009) 104503.

S. Chandrasekhar, “Hydrodynamic and Hydromagnetic Stability”, Dover Publications, Inc. (2013).

N. Papamarkos, B. Mertzios & G. Vachtsevanos, “On the optimum design of symmetric two-dimensional iir digital filters with coefficients of finite word length”, International journal of circuit theory and applications 14 (1986) 339.

G. Rana & R. Chand, “Stability analysis of double-diffusive convection of rivlin-ericksen elastico-viscous nanofluid saturating a porous medium: a revised model”, Forschung im Ingenieurwesen 79 (2015) 87.

R. Chand, “Nanofluid Technologies and Thermal Convection Techniques”, IGI Global (2017).

R. Singh, V. K. Tyagi & J. Bishnoi, “A study of non-newtonian nanofluid saturated in a porous medium based on modified darcy-maxwell model”, Cognitive Informatics and Soft Computing: Proceeding of CISC (2022) 241.

E. Lapwood, “Convection of a fluid in a porous medium”, Mathematical Proceedings of the Cambridge Philosophical Society 44 (1948) 508.

D. A. Nield & A. Bejan, “Convection in Porous Media”, Springer 3 (2006).

D. Nield & A. Kuznetsov, “Onset of convection with internal heating in a porous medium saturated by a nanofluid”, Transport in porous media 99 (2013) 73.

D. Nield & C. T. Simmons, “A brief introduction to convection in porous media”, Transport in Porous Media 130 (2019) 237.

D. Y. Tzou, “Thermal instability of nanofluids in natural convection”, International Journal of Heat and Mass Transfer 51 (2008) 2967.

D. Tzou, “Instability of nanofluids in natural convection”, Journal of Heat Transfer 130 (2008).

D. Nield & A. V. Kuznetsov, “Thermal instability in a porous medium layer saturated by a nanofluid”, International Journal of Heat and Mass Transfer 52 (2009) 5796.

D. Nield & A. V. Kuznetsov, “The onset of convection in a horizontal nanofluid layer of finite depth”, European Journal of Mechanics-B/Fluids 29 (2010) 217.

A. Kuznetsov & D. Nield, “Thermal instability in a porous medium layer saturated by a nanofluid: Brinkman model”, Transport in Porous Media 81 (2010) 409.

L. J. Sheu, “Thermal instability in a porous medium layer saturated with a viscoelastic nanofluid”, Transport in Porous Media 88 (2011) 461.

R. Chand & G. Rana, “Thermal instability of rivlin–ericksen elasticoviscous nanofluid saturated by a porous medium”, Journal of fluids engineering 134 (2012).

R. Chand & G. C. Rana, “Hall effect on thermal instability in a horizontal layer of nanofluid saturated in a porous medium”, International Journal of Theoretical and Applied Multiscale Mechanics 3 (2014) 58.

R. Chand & D. Puigjaner, “Thermal instability analysis of an elasticoviscous nanofluid layer”, Engineering Transactions 66 (2018) 301.

M.Ramanuja, J. Kavitha, A. Sudhakar & N. Radhika, “Study of MHD SWCNT-Blood Nanofluid Flow in Presence of Viscous Dissipation and Radiation Effects through Porous Medium”, Journal of the Nigerian Society of Physical Sciences (2023) 1054.

S. Kaothekar, “Thermal instability of partially ionized viscous plasma with hall effect flr corrections flowing through porous medium”, Journal of Porous Media 21 (2018).

R. Chand & S. Kango, “Thermal instability of oldroydian visco-elastic nanofluid in a porous medium for more realistic boundary conditions”, Special Topics & Reviews in Porous Media: An International Journal 12 (2021).

R. Sharma, “Effect of rotation on thermal instability of a viscoelastic fluid”, Acta Physica Academiae Scientiarum Hungaricae 40 (1976) 11.

D. Yadav, G. Agrawal & R. Bhargava, “Thermal instability of rotating nanofluid layer”, International Journal of Engineering Science 49 (2011) 1171.

R. Chand & G. Rana, “On the onset of thermal convection in rotating nanofluid layer saturating a darcy–brinkman porous medium”, International Journal of Heat and Mass Transfer 55 (2012) 5417.

R. Chand, G. Rana, A. Kumar & V. Sharma, “Thermal instability in a layer of nanofluid subjected to rotation and suspended particles”, Research Journal of Science and Technology 5 (2013) 2.

S. Govender, “Thermal instability in a rotating vertical porous layer saturated by a nanofluid”, Journal of Heat Transfer 138 (2016).

R. Chand, D. Yadav & G. C. Rana, “Thermal instability of couple-stress nanofluid with vertical rotation in a porous medium”, Journal of Porous Media 20 (2017).

D. Yadav, R. Bhargava, G. Agrawal, G. S. Hwang, J. Lee & M. Kim,

“Magneto-convection in a rotating layer of nanofluid”, Asia-Pacific Journal of Chemical Engineering 9 (2014) 663.

R. Chand, D. Yadav & G. Rana, “Electrothermo convection in a horizontal layer of rotating nanofluid”, International Journal of Nanoparticles 8 (2015) 241.

R. Chand & G. Rana, “Oscillating convection of nanofluid in porous medium”, Transport in Porous Media 95 (2012) 269.

P. K. Gautam, G. C. Rana & H. Saxena, “Stationary convection in the electrohydrodynamic thermal instability of jeffrey nanofluid layer saturating a porous medium: free-free, rigid-free, and rigid-rigid boundary conditions”, Journal of Porous Media 23 (2020).

G. C. Rana, “Effects of rotation on jeffrey nanofluid flow saturated by a porous medium”, Journal of Applied Mathematics and Computational Mechanics 20 (2021) 17.

P.L. Sharma, M. Kapalta, A. Kumar, D. Bains, S. Gupta, & P. Thakur. ”Electrohydro dynamics convection in dielectric rotating Oldroydian nanofluid in porous medium”, Journal of the Nigerian Society of Physical Sciences (2023) 1231.

G. Pradhan, P. Samal & U. Tripathy, “Thermal stability of a fluid layer in a variable gravitational field”, Indian J. pure appl. Math 20 (1989) 736.

S. M. Alex, P. R. Patil & K. Venkatakrishnan, “Variable gravity effects on thermal instability in a porous medium with internal heat source and inclined temperature gradient”, Fluid Dynamics Research 29 (2001) 1.

B. Straughan, “The Energy Method, Stability, and Nonlinear Convection”, Springer Science & Business Media 91 (2013).

R. Chand, G. Rana & S. Kumar, “Variable gravity effects on thermal instability of nanofluid in anisotropic porous medium”, International Journal of Applied Mechanics and Engineering 18 (2013) 631.

D. Yadav, “Numerical investigation of the combined impact of variable gravity field and throughflow on the onset of convective motion in a porous medium layer”, International communications in heat and mass transfer 108 (2019) 104274.

A. Mahajan & V. K. Tripathi, “Effects of spatially varying gravity, temperature and concentration fields on the stability of a chemically reacting fluid layer”, Journal of Engineering Mathematics 125 (2020) 23.

D. Surya & A. Gupta, “Thermal instability in a liquid layer with permeable boundaries under the influence of variable gravity”, European Journal of Mechanics-B/Fluids 91 (2022) 219.

D. Yadav, “Effects of rotation and varying gravity on the onset of convection in a porous medium layer: a numerical study”, World Journal of Engineering 17 (2020) 785.

S. Shekhar, R. Ragoju & D. Yadav, “The effect of variable gravity on rotating rayleigh–Benard convection in a sparsely packed porous layer”,´ Heat Transfer 51 (2022) 4187.

R. Chand, G. Rana & S. Kango, “Effect of variable gravity on thermal 10

instability of rotating nanofluid in porous medium”, FME Transactions 43 (2015) 62.

Published

2023-05-20

How to Cite

Thermal instability of rotating Jeffrey nanofluids in porous media with variable gravity. (2023). Journal of the Nigerian Society of Physical Sciences, 5(2), 1366. https://doi.org/10.46481/jnsps.2023.1366

Issue

Volume 5, Issue 2, May 2023

Section

Original Research
Copyright & Licensing

Copyright (c) 2023 Pushap Lata Sharma, Deepak Bains, Pankaj Thakur

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Thermal instability of rotating Jeffrey nanofluids in porous media with variable gravity. (2023). Journal of the Nigerian Society of Physical Sciences, 5(2), 1366. https://doi.org/10.46481/jnsps.2023.1366