Constructive approach and randomization of a two-parameter chaos system for securing data

Authors

  • Olalekan Taofeek Wahab Department of Mathematics and Statistics, Kwara State University, Malete, Nigeria
  • Salaudeen Alaro Musa Department of Mathematics and Statistics, Kwara State University, Malete, Nigeria
  • AbdulAzeez Kayode Jimoh Department of Mathematics and Statistics, Kwara State University, Malete, Nigeria
  • Kazeem Adesina Dauda Department of Mathematics and Statistics, Kwara State University, Malete, Nigeria

Keywords:

Two-parameter chaos system, Cryptosystems, Encryption algorithm, Lipschitz map, Pseudo-contractive operator

Abstract

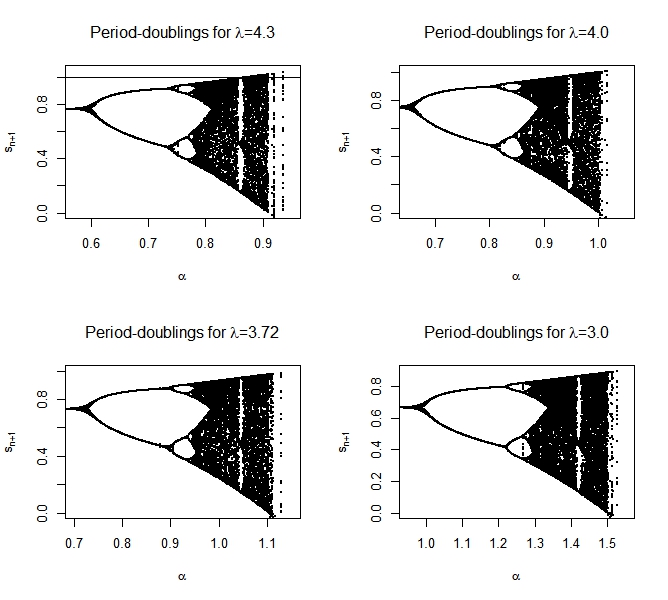
Secure communication techniques are important due to the increase in the number of technology users across the world. Likewise, a more random encryption algorithm suitable to secure data from unauthorised users is highly expected. This paper proposes a two-parameter nonlinear chaos map that is sensitive to the trio seed (s0, \alpha, \lambda) and has better information encryption. We introduce the parameter \alpha to linearise the conventional chaos system, which in turn brings a delay in the cryptosystems. The delay is a phenomenon that changes the chaotic features of a system. A small delay in the system leads to more aperiodicity and the unpredictability of the chaotic attractions. We normalise the new chaos map and use the Lipschitz and pseudo-contractive operators to obtain its irregularity region in Hilbert spaces. We also analyse the chaos map in terms of trajectory, Lyapunov exponent, complexity, and information entropy. Results obtained show that the new chaos map has a wide chaotic range and better statistical properties. It also maintains low complexity due to its linearity and produces more key spaces than most existing chaotic maps.

Dimensions

C. Li, D. Lin, B. Feng, J. Lu & F. Hao, “Cryptanalysis of a chaotic image encryption algorithm based on information entropy”, IEEE Access 6 (2008) 75834. https://doi.org/10.1109/ACCESS.2018.2883690.

M. Andrecut, “Logistic map as a random number generator”, Int. J. Mod. Phys. B. 12 (1998) 921. https://doi.org/10.1142/S021797929800051X.

W. Zhao, Z. Chang, C. Ma, C. Ma & Z. Shen, “A pseudorandom number generator based on the chaotic map and quantum random walks”, Entropy 25 (2023) 166. https://doi.org/10.3390/e25010166.

H. Hu, L. Liu & N. Ding, “Pseudorandom sequence generator based on the Chen chaotic system”, Comput. Phys. Commun. 184 (2013) 765. https://doi.org/10.1016/j.cpc.2012.11.017.

V. Patidar, K. K. Sud & N. K. Pareek, “A pseudo random bit generator based on chaotic logistic map and its statistical testing”, Informatica 33 (2019) 441. https://www.informatica.si/index.php/informatica/article/view/261/258.

J. Teh, W. Teng, A. Samsudin & J. Chen, “A post-processing method for true random number generators based on hyperchaos with applications in audio-based generators”, Frontiers of Computer Science 14 (2020) 146405. https://doi.org/10.1007/s11704-019-9120-2.

X. Y.Wang & L. Yang, “Design of pseudo-random bit generator based on chaotic maps”, Int. J. Mod. Phys. 26 (2012) 1250208. https://doi.org/10.1142/S0217979212502086.

Y. Wang, Z. Liu, J. Ma & H. He, “A pseudo-random number generator based on piecewise logistic map”, Nonlinear Dyn. 83 (2016) 2373. https://doi.org/10.1007/s11071-015-2488-0.

Y. Wu, G. Yang, H. Jin & J. P. Noonan, “Image encryption using the two-dimensional logistic chaotic map”, Journal of Electronic Imaging 21 (2012) 013014. https://doi.org/10.1117/1.JEI.21.1.013014.

L. Xu, Z. Li, J. Li & W. Hua, “A novel bit-level image encryption algorithm based on chaotic maps”, Opt. Lasers Eng. 78 (2016) 17. https://doi.org/10.1016/j.optlaseng.2015.09.007.

P. Li, Z. Li, W. A. Halang & G. Chen, “A novel multiple pseudo random bits generator based on spatiotemporal chaos”, IFAC Proc. 38 (2005) 1085. https://doi.org/10.3182/20050703-6-CZ-1902.00837.

A. S. Mansingka, A. G. Radwan & K. N. Salama, “Fully digital 1-D, 2-D and 3-D multiscroll chaos as hardware pseudo random number generators”, In Proceedings of the International Midwest Symposium on Circuits and Systems (MWSCAS), Boise, ID, USA, 2012, pp. 1180-1183. https://doi.org/10.1109/MWSCAS.2012.6292236.

Y. Mao, L. Cao & W. Liu, “Design and FPGA implementation of a pseudo-random bit sequence generator using spatiotemporal chaos”, In Proceedings of the International Conference on Communications, Circuits and Systems, Guilin, China, 2006, pp. 2114-2118. https://doi.org/10.1109/ICCCAS.2006.284916.

H. T. Yang, J. R. Huang & T. Y. Chang, “A chaos-based fully digital 120 MHz pseudo random number generator”, In Proceedings of the IEEE Asia-Pacific Conference on Circuits and Systems, Tainan, Taiwan, 2004, pp. 357-360. https://doi.org/10.1109/APCCAS.2004.1412769.

J. Liu, Z. Liang, Y. Luo, L. Cao, S. Zhang, Y. Wang & S. Yang, “A hardware pseudo-random number generator using stochastic computing and logistic map”, Micromachines 12 (2021) 31. https://doi.org/10.3390/mi12010031.

L. Wang & H. Cheng, “Pseudo-random number generator based on logistic chaotic system”, Entropy 21 (2019) 960. https://doi.org/10.3390/e21100960.

S. Li, W. Ding, B. Yin, T. Zhang & Y. Ma, “A novel delay linear coupling logistics map model for color image encryption”, Entropy 20 (2018) 463. https://doi.org/10.3390/e20060463.

V. Berinde, Iterative approximation of fixed points, (2nd ed.) Springer-Verlag, Berlin Heidelberg, 2007. https://doi.org/10.1007/978-3-540-72234-2.

O. T. Wahab, R. O. Olawuyi, K. Rauf & I. F. Usamot, “Convergence rate of some two-step iterative schemes in Banach spaces”, Journal of Mathematics 2016 (2016) 9641706. https://doi.org/10.1155/2016/9641706.

H. Akaike, “Akaike’s information criterion”, In Lovric M. (eds) International Encyclopedia of Statistical Science, Springer, Berlin, Heidelberg, 2011. https://doi.org/10.1007/978-3-642-04898-2_110.

G. Schwarz, “Estimating the dimension of a model”, Annals of Statistics 6 (1978) 461. https://doi.org/10.1214/aos/1176344136.

G. Alvarez & S. Li, “Some basic cryptographic requirements for chaosbased cryptosystems”, Int. J. Bifurc. Chaos. 16 (2006) 2129. https://doi.org/10.1142/S0218127406015970.

F. O¨ zkaynak, “Brief review on application of nonlinear dynamics in image encryption”, Nonlinear Dyn. 92 (2018) 305. https://doi.org/10.1007/s11071-018-4056-x.

Published

2024-05-21

How to Cite

Constructive approach and randomization of a two-parameter chaos system for securing data. (2024). Journal of the Nigerian Society of Physical Sciences, 6(2), 1747. https://doi.org/10.46481/jnsps.2024.1747

Issue

Volume 6, Issue 2, May 2024

Section

Mathematics & Statistics
Copyright & Licensing

Copyright (c) 2024 Olalekan Taofeek Wahab, Salaudeen Alaro Musa, AbdulAzeez Kayode Jimoh, Kazeem Adesina Dauda

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Constructive approach and randomization of a two-parameter chaos system for securing data. (2024). Journal of the Nigerian Society of Physical Sciences, 6(2), 1747. https://doi.org/10.46481/jnsps.2024.1747