Analysis of a fractional order climate model due to excessive emission and accumulation of carbon dioxide in the atmosphere

Authors

  • Peter Urane Achimugwu Pan African University Institute for Basic Sciences, Technology and Innovation, Nairobi, Kenya
  • Mathew Ngugi Kinyanjui Department of Pure and Applied Mathematics, Jomo Kenyatta University of Agriculture and Technology, Juja, Kenya
  • David Mumo Malonza Department of Mathematics and Actuarial Science, South Eastern Kenya University, Kitui, Kenya

Keywords:

Climate change, Fractional order, Carbon dioxide, Excessive emission

Abstract

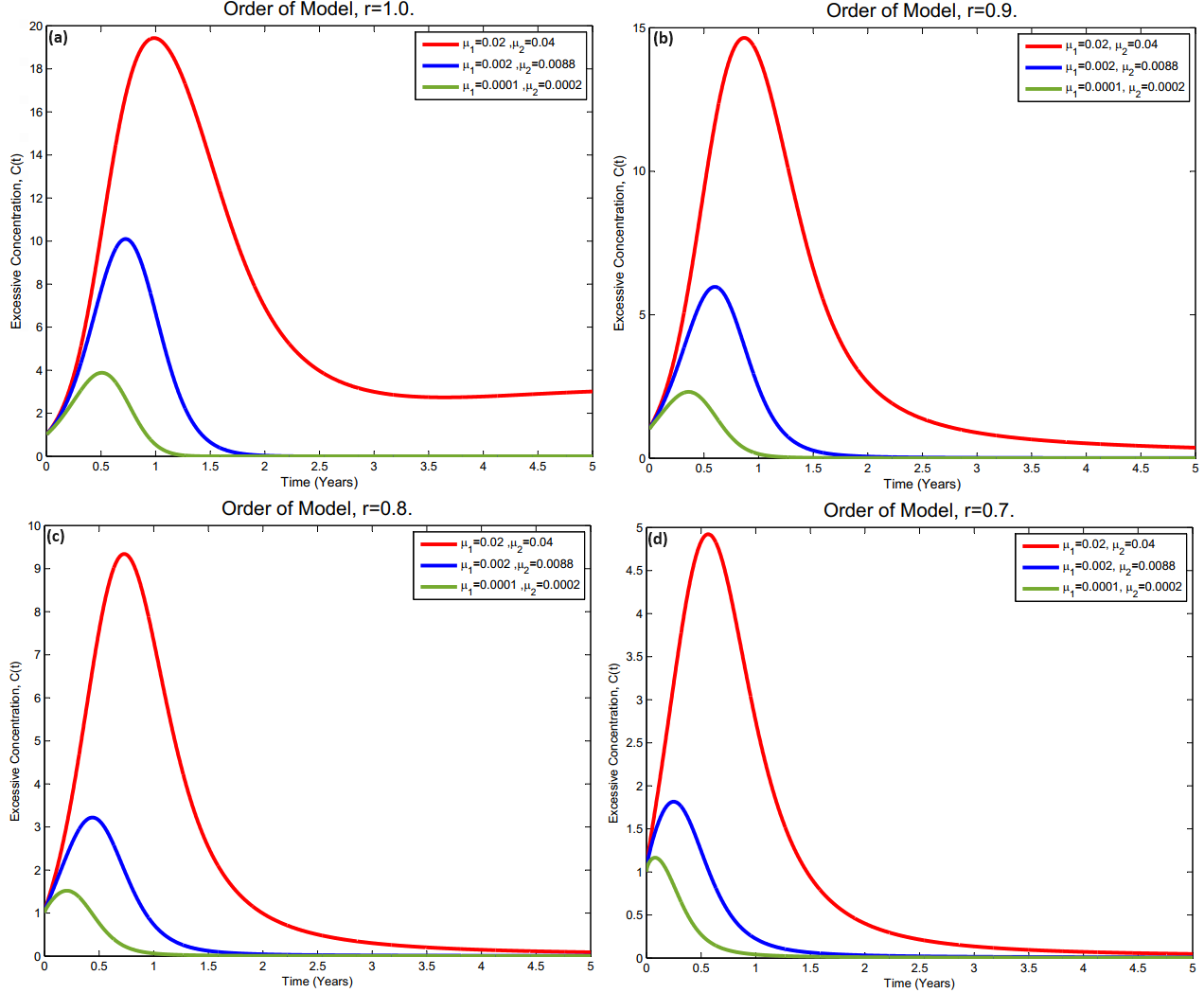
The devastating consequences of climate change on our planet cannot be taken lightly. Greenhouse gas emissions due to the various activities of the increasing human population are solely responsible for this change. The chief and most significant of these gases is carbon dioxide. A fractional-order model of five compartments is considered. The uniqueness and existence, the positivity, and the boundedness of the model solution are established. The equilibrium points of the model are given. By formulating different Lyapunov functions, the global stability of the four equilibrium points was determined. The numerical simulation of the model was done using the Predict-Evaluate-Correct-Evaluate method of Adam-Bashforth-Moulton by considering four different orders of 0.7, 0.8, 0.9, and 1.0. According to the results, excessive concentrations of carbon dioxide in the atmosphere can be reduced by the joint employment of mitigation measures.

Dimensions

J. Song, Q. Song, D. Zhang, Y. Lu,& L. Luan, “Study on infuencing factors of carbon emissions from energy consumption of shandong province of china from 1995 to 2012”, The Scientific World Journal (2014) 1. https://doi.org/10.1155/2014/684796

C. Zhu & D. Gao, “A research on the factors influencing carbon emission of transportation industry in “ the belt and road initiative” countries based on panel data”, Energies 12 (2019)1. https://doi.org/10.3390/en12122405

K. Peterson, “Explaining human influences on carbon dioxide emissions across countries”, Honors Projects 100 (2009). https://digitalcommons.iwu.edu/econ honproj/100

F. Vadillo, “On the walker’s model for the carbon dioxide in the earth’s atmosphere”, Journal of Environmental Protection 10 (2019) 48. https: //doi.org/10.4236/jep.2019.101004

S. Banaji, “Nitrogen dioxide, carbon monoxide, natural and anthropomorphic effects, and earth’s changing climate”, Atmospheric and Climate Sciences, 13 (2023) 62. https://doi.org/10.4236/acs.2023.131005

O. O. Ayeleru, H. U. Modekwe, O. R. Onisuru, C. R. Ohoro, C. A. Akinnawo, & P. A. Olubambi, “Adsorbent technologies and applications for carbon capture, and direct air capture in environmental perspective and sustainable climate action”, Sustainable Chemistry for Climate Action 3 (2023) 100029. https://doi.org/10.1016/j.scca.2023.100029

V. Moutinho, M. Madaleno, R. Inglisi-Lotz, & E. Dogan, “Factors affecting carbon dioxide emissions in top countries on renewable energies : A LMDI decomposition application”, Renewable and Sustainable Energy Reviews 90 (2018) 605. https://doi.org/10.1016/j.rser.2018.02.009

D. K. Almutairi, M. A. Abdoon, S. Y. M. Salih, S. A. Elsamani, F. E. L. Guma, & M. Berir, “Modeling and analysis of a fractional visceral leishmaniosis with caputo and caputo-fabrizio derivatives”, J. Nig. Soc. Phys. Sci. 5 (2023)1453. https://doi.org/10.46481/jnsps.2023.1453

S. A. Abdulahi, A. G. Habib & N. Hussaini, “Mathematical model of in-host dynamics of snakebite envenoming”, J. Nig. Soc. Phys. Sci. 4 (2022)193. https://doi.org/10.46481/jnsps.2022.548.

A. Umar, S. Musa & A. A. Agada, “Analysis of varroosis model in honeybee colony with interventions”, African Scientific Reports 2 (2023) 98. https://doi.org/10.46481/asr.2023.2.2.98

S. Mandal, M. S. I Sonia Akter, & M. H. A. Biswas, “A mathematical model to investigate the frequent impact of global warming on coastal lives”, In Procceedings of the International Conference on Industrial and Mechanical Engineering and Operations Management, Dhaka, Bangladesh, 2020. https: //www.google.com/url?sa=t&rct=j&q=&esrc=s&source=web&cd= &ved=2ahUKEwj78IbslemBAxW33AIHHUP4A6YQFnoECAoQAQ& url=http%3A%2F%2Fwww.ieomsociety.org%2Fimeom%2F140.pdf& usg=AOvVaw3aFsY0H0-GVVyDsHj41L9n&opi=89978449

A. K. Misra, M. Verma & E. Venturino, “Modelling the control of atmospheric carbon dioxide through reforestation:effect of time delay. Model”, Earth Syst. Environ 1 (2015) 1. https://doi.org/10.1007/ s40808-015-0028-z

S. Mondal, M. Biswas & N. Bairagi, “Local and global dynamics of a fractional-order predator-prey system with habitat complexity and the corresponding discretized fractional-order system”, Journal of Applied Mathematics and Computing 63 (2020) 1. https://doi.org/10.1007/ s12190-020-01319-6

B. F. Etin-Osa & I. M. Azubike, “Parametric sensitivity analysis of a mathematical model of the effect of carbon dioxide on the climate change”, Applied and Computational Mathematics 9 (2020) 96. 10. 11648/j.acm.20200903.16

K. R. Gandhi & M. R. Tailor, “Solution of problems arising due global warming and climate change in the modelling process”, International Journal for Research in Applied Science and Engineering Technology 8 (2020) 1. https://www.ijraset.com

S. Sundar, R. Naresh, B. Dubey & J. B. Shukla, “Modeling the removal of cement dust particles from a chimney by water sprays and green plantation”, International e-Journal of Mathematical Modeling and Analysis of Complex Systems: Nature and Society 1 (2015) 52.

A. H. Teru & P. R. Koya, “Mathematical modelling of deforestation of forested area due to lack of awareness of human and its conservation”, Mathematical Modelling and Applications 5 (2020) 94. http://dx.doi.org/10.11648/j.mma.20200502.15

P. U. Achimugwu, M. N. Kinyanjui & D.M. Malonza, “Mitigation of climate change due to excessive carbon dioxide emission and accumulation: A mathematical model approach”, Commun. Math. Biol. Neurosci. 70 (2023)1. https://doi.org/10.28919/cmbn/8027

R. Almeida, “Dynamical analysis of a fractional sir model with treatment and quarantine”, Chaotic Modelling & Simulation (CMSIM) 2 (2020) 115. https://www.researchgate.net/publication/341407213 Dynamical analysis of a fractional SIR model with treatment and quarantine

N. Yuan, Z. Fu, & S. Liu, “Extracting climate memory using fractional integrated statistical model: A new perspective on climate prediction”, Scientific Reports 4 (2014) 1. https://doi.org/10.1038/srep06577

P. Kumar, V. Govindaraj, V. S. Ertuk, & M. S. Mohamed, “Effects of greenhouse gases and hypoxia on the population of aquatic species: A fractional mathematical model”, Advances in Continuous and Discrete Models 31 (2022) 1. https://doi.org/10.1186/s13662-022-03679-8

S. C. Eze & M. O. Oyesanya, “Fractional order on the impact of climate change with dominant earth’s fluctuations”, Math.Clim.Weather Forecast, 5 (2019) 1. http://dx.doi.org/10.1515/mcwf-2019-0001

W. Xie, W-Z. Wu, C. Liu, T. Zhang & Z. Dong, “Forecasting fuel combustion-related carbon dioxide emissions by a novel continuous fractional nonlinear grey bernoulli model with grey wolf optimizer”, Environmental Science and Pollution Research 28 (2021) 1. https://doi.org/ 10.1007/s11356-021-12736-w

E. Ilhan, P. Veeresha & H. M. Baskonus, “Fractional approach for a mathematical model of atmospheric dynamics of carbon dioxide gas with an efficient method”, Chaos, Solitons & Fractals 152 (2021) 111347. https://doi.org/10.1016/j.chaos.2021.111347

R. Ozarslan & Y. Sekerci, “Fractional order oxygen-plankton system under climate change”, Chaos: An Interdisciplinary Journal of Nonlinear Science 30 (2020) 1. https://doi.org/10.1063/1.5129766

S. Qureshi & A. Yusuf, “Mathematical modeling for the impacts of deforestation on wildlife species using caputo differential operator”, Chaos, Solitons & Fractals 126 (2019) 32. https://doi.org/10.1016/j.chaos.2019. 05.037

Y. Sekerci & R. Ozarslan, “Oxygen-plankton model under the effect of global warming with nonsingular fractional order”, Chaos, Solitons & Fractals 132 (2020) 1. https://doi.org/10.1016/j.chaos.2019.109532

A. Din, F. M. Khan, Z. U. Khan, A. Yusuf, & T. Munir, “The mathematical study of climate change model under nonlocal fractional derivative”, Partial Differential Equations in Applied Mathematics 5 (2022) 1. https://doi.org/10.1016/j.padiff.2021.100204

J. A. T. Machado & A. M. Lopes, “Dynamical analysis of the global warming”, Mathematical Problems in Engineering 2012 (2012) 1. https: //doi.org/10.1155/2012/971641

Z. Xu, L. Liu & L. Wu, “Forecasting the carbon dioxide emissions in 53 countries and regions using a non-equigap grey model”, Environmental Science and Pollution Research 28 (2021) 15659. https://doi.org/10.1007/ s11356-020-11638-7

M. F. Elettreby, M. Nabil & A.A. Al-Raezah, “Dynamical analysis of a prey-predator fractional order model”, Journal of Fractional Calculus and Applications 8 (2017) 237.http://fcag-egypt.com/Journals/JFCA/

A. Suryanto, I. Darti, H. S. Panigoro & A. Kilicman, “A fractionalorder predator-prey model with ratio-dependent functional response and linear harvesting”, Mathematics 7 (2019) 1.https://doi.org/10.3390/math7111100

M. F. Carfora & I. Torcicollo, “A fractional-in-time prey-predator model with hunting cooperation: Qualitative analysis, stability and numerical approximations”, Axioms 10 (2021) 1. https://doi.org/10.3390/ axioms10020078

C. Vargas-De-Leon, “Volterra-type lyapunov functions for fractionalorder epidemic systems”, Commun. Nonlinear Sci Numer Simulat 24 (2015) 75. https://doi.org/10.1016/j.cnsns.2014.12.013

N. Aguila-Camacho, M. A. Duarte-Mermoud, & J. A. Gallegos, ” Lyapunov functions for fractional order systems”, Commun Nonlinear Sci Numer Simulat 19 (2014) 2951. https://doi.org/10.1016/j.cnsns.2014.01. 022

I. Ahmed, I. A. Baba, A. Yusuf, P. Kumam, & W. Kumam, ” Analysis of caputo fractional-order model for covid-19 with lockdown”, Advances in Difference Equations 2020 (2020) 394. https://doi.org/10.1186/ s13662-020-02853-0

M. R. Lemnaouar, M. Khalfaoui, Y. Louartassi & I. Tolaimate, “Fractional order prey-predator model with infected predators in the presence of competition and toxicity”, Math. Model. Nat. Phenom. 15 (2020) 1. https://doi.org/10.1051/mmnp/2020002

H. S. Panigoro, A. Suryanto, W. M. Kusumawinahyu & I. Darti, “Global stability of a fractional-order gause-type predator-prey model with threshold harvesting policy in predator”, Commun. Math. Biol. Neurosci. 63 (2021) 1. https://doi.org/10.28919/cmbn/6118

J. A. Akingbade & B. S. Ogundare, “Boundedness and stability properties of solutions of mathematical model of measles”, TAMKANG JOURNAL OF MATHEMATICS 52 (2021) 91. https://doi.org/10.5556/j.tkjm. 52.2021.3367

M. A. Aba Oud, A. Ali, H. Alrabaiah, S. Ullah, M. A. Khan, & S. Islam, “A fractional order mathematical model for covid-19 dynamics with quarantine, isolation, and environmental viral load”, Advances in Difference Equations 106 (2021) 1. https://doi.org/10.1186/s13662-021-03265-4

A. Rehman, R. Singh, T. Abdeljawad, E. Okyere, & L. Guran, “Modeling,analysis and numerical solution to malaria fractional model with temporary immunity and relapse”, Advances in Difference Equations 390 (2021) 1. https://doi.org/10.1186/s13662-021-03532-4

G. T. Tilahun, W. A. Woldegerima & N. Mohammed, “A fractional order model for the transmission dynamics of hepatitis b virus with twoage structure in the presence of vaccination”, Arab Journal of Basic and Applied Sciences 28 (2021) 87. https://doi.org/10.1080/25765299.2021. 1896423

S. Sundar, “An ecological type nonlinear model for the removal of carbon dioxide from the atmosphere by introducing liquid species”, Combinatorial Ecology and Software 3 (2013) 33.

http://www.iaees.org/publications/journals/ces/articles/2013-3%282%29/an-nonlinear-model-for-removal-of-carbon-dioxide.pdf

P. Panja, “Is the forest biomass a key regulator of global warming? A mathematical modelling study”, Geology, Ecology, and Landscapes 2020 (2020) 1. https://doi.org/10.1080/24749508.2020.1752021

M. Kizito & J. Tumwiine, “Mathematical model of treatment and vaccination interventions of pneumococcal pneumonia infection dynamics”, Journal of Applied Mathematics 2018 (2018) 1. https://doi.org/10.1155/2018/2539465

E. Mayanja, L. S. Luboobi, J. Kasozi, & R. N. Nsubuga, “Mathematical modelling of hiv-hcv coinfection dynamics in absence of therapy”, Computational and Mathematical Methods in Medicine 2020 (2020) 1. https://doi.org/10.1155/2020/2106570

I. Zada, M. N. Jan, N. Ali, D. Alrowail, K. S. Nisar, & G. Zaman, “ Mathematical analysis of hepatitis B epidemic model with optimal control”, Advances in Difference Equations 451 (2021) 1. https://doi.org/10.1186/ s13662-021-03607-2

S. B. Hsu, “A survey of constructing lyapunov functions for mathematical models in population biology”, Taiwanese Journal of Mathematics 9 (2005) 151. https://www.jstor.org/stable/43833596

S. Guo & W. Jiang, “Global stability and hopf bifurcation for gause-type predator-prey system”, Journal of Applied Mathematics 2012 (2012) 1. https://doi.org/10.1155/2012/260798

P. Andayani, “Global stability analysis on a predator-prey model with omnivores”, Applied Mathematical Sciences 9 (2015) 1771. http://dx.doi. org/10.12988/ams.2015.5120

C. Liu, Z. Wang, & B. Weng, “Dynamical analysis of fractional-order holling type-ii food chain model”, Mathematical Biosciences and Engineering 18 (2021) 5221. https://doi.org/10.3934/mbe.2021265.

J. Baranowski, M. Zagorowska, W. Bauer, T. Dziwinski, & P. Piatek, “Applications of direct lyapunov method in caputo non-integer order systems”, ELEKTRONIKA IR ELEKTROTECHNIKA 21 (2015) 1. https://dx.doi.org/10.5755/j01.eee.21.2.11505

S. Rosa & D. F. M. Torres, “Numerical fractional optimal control of respiratory syncytial virus infection in octave/matlab”, Mathematics 11 (2023) 1511. https://doi.org/10.3390/math11061511

A. Altamirano-Fernandez, A. Rojas-Palma & S. Espinoza-Meza, “ Optimal management strategies to maximize carbon capture in forest plantations: A case study with pinus radiata d.don”, Forests 14 (2023) 1.

https://doi.org/10.3390/f14010082

Published

2023-10-18

How to Cite

Analysis of a fractional order climate model due to excessive emission and accumulation of carbon dioxide in the atmosphere. (2023). Journal of the Nigerian Society of Physical Sciences, 5(4), 1749. https://doi.org/10.46481/jnsps.2023.1749

Issue

Volume 5, Issue 4, November 2023

Section

Original Research
Copyright & Licensing

Copyright (c) 2023 Peter Urane Achimugwu, Mathew Ngugi Kinyanjui, David Mumo Malonza

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Analysis of a fractional order climate model due to excessive emission and accumulation of carbon dioxide in the atmosphere. (2023). Journal of the Nigerian Society of Physical Sciences, 5(4), 1749. https://doi.org/10.46481/jnsps.2023.1749