On sentinel method of one-phase Stefan problem

https://doi.org/10.46481/jnsps.2023.1772

Authors

  • Merabti Nesrine Lamya Department of Mathematics and Computer Science, University of Oum El Bouaghi, Oum El Bouaghi 04000, Algeria
  • Iqbal M. Batiha Department of Mathematics, Al Zaytoonah University, Amman 11733, Jordan | Nonlinear Dynamics Research Center (NDRC), Ajman University, Ajman 346, UAE
  • Imad Rezzoug Department of Mathematics and Computer Science, University of Oum El Bouaghi, Oum El Bouaghi 04000, Algeria
  • Adel Ouannas Department of Mathematics and Computer Science, University of Oum El Bouaghi, Oum El Bouaghi 04000, Algeria
  • Taki-Eddine Ouassaeif Department of Mathematics and Computer Science, University of Oum El Bouaghi, Oum El Bouaghi 04000, Algeria

Keywords:

Sentinel method, Approximate controllability, Stefan’s problem

Abstract

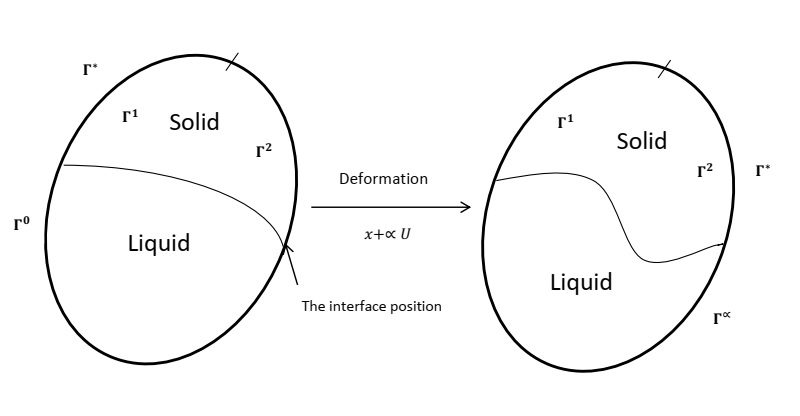
This paper is interested in studying the one-phase Stefan problem. For this purpose, we use the nonlinear sentinel method, which relies typically on the approximate controllability and the Fanchel-Rockafellar duality of the minimization problem, to prove the existence and uniqueness of a solution to this problem. In particular, our research focuses on the application of the nonlinear sentinel method to the single-phase Stefan problem. This approach aids in identifying an unspecified boundary section within the domain undergoing a liquid-solid phase transition. We track the evolution of the temperature profile in the liquid-solid material and the corresponding movement of its interface over time. Eventually, the local convergence used for the iterative numerical scheme is demonstrated.

Dimensions

A. Hioual, A. Ouannas, T. E. Oussaeif, G. Grassi, I. M. Batiha & S. Momani, “On variable-order fractional discrete neural networks: solvability and stability”, Fractal and Fractional, 6 (2022), 119. https://doi.org/10. 3390/fractalfract6020119.

A. Hioual, A. Ouannas, G. Grassi & T. E. Oussaeif, “Nonlinear nabla variable-order fractional discrete systems: asymptotic stability and application to neural networks”, Journal of Computational and Applied Mathematics 423 (2023) 114939. https://doi.org/10.1016/j.cam.2022.114939.

A. Hioual, T. E. Oussaeif, A. Ouannas, G. Grassi, I. M. Batiha & S. Momani, “New results for the stability of fractional-order discretetime neural networks”, Alexandria Engineering Journal 61 (2022) 10359. https://doi.org/10.1016/j.aej.2022.03.062.

A. Hioual, A. Ouannas, T. E. Oussaeif & Z. Laouar, On fractional variable-order neural networks based on the Caputo derivative, Proceedings of the 4th International Conference on Pure and Applied Mathematics, Van Yuz¨ unc¨ u Yıl University, Van, TURKEY, 2022, pp. 103–104.¨ http://icpam.yyu.edu.tr/abstractbook.pdf.

H. Qawaqneh, M. S. M. Noorani, H. Aydi, A. Zraiqat & A. H. Ansari, “On fixed point results in partial-metric spaces”, Journal of Function Spaces, 2021 (2021), 8769190. https://doi.org/10.1155/2021/8769190.

B. Abdelwahb, T. E. Oussaeif, A. Ouannas, K. M. Saad, H. Jahanshahi, A. Diar, A. M. Aljuaid & A. A. Aly, “A nonlinear fractional problem with a second kind integral condition for time-fractional partial diferential equation”, Journal of Function Spaces, 2022 (2022), 2913587. https://doi.

org/10.1155/2022/2913587.

N. Djenina, A. Ouannas, I. M. Batiha, G. Grassi, T. E. Oussaeif & S. Momani, “A novel fractional-order discrete SIR model for predicting COVID-19 behavior”, Mathematics, 10 (2022), 2224. https://doi.org/10. 3390/math10132224.

N. Djenina, A. Ouannas, T. E. Oussaeif, G. Grassi, I. M. Batiha, S. Momani & R. B. Albadarneh, “On the stability of incommensurate h-nabla fractional-order diference systems”, Fractal and Fractional, 6 (2022), 158.

https://doi.org/10.3390/fractalfract6030158.

T. E. Oussaeif, B. Antara, A. Ouannas, I. M. Batiha, K. M. Saad, H. Jahanshahi, A. M. Aljuaid & A. A. Aly, “Existence and uniqueness of the solution for an inverse problem of a fractional difusion equation with integral condition”, Journal of Function Spaces, 2022 (2022), 7667370. https://doi.org/10.1155/2022/7667370.

Z. Chebana, T. E. Oussaeif, A. Ouannas & I. Batiha, “Solvability of Dirichlet problem for a fractional partial differential equation by using energy inequality and Faedo-Galerkin method”, Innovative Journal of Mathematics, 1 (2022), 34. https://doi.org/10.55059/ijm.2022.1.1/4.

I. Rezzoug & A. Ayadi, Etude the´orique et nume´rique des proble`mes d’identification des syste`mes gouverne´s pardes e´quations aux de´rive´es partielles, PhD thesis, Department of Mathematics and Computer Science, Universite d’Oum El Bouaghi, d’Oum El´ Bouaghi, Algeria, 2015. https://theses.hal.science/tel-03169460/file/ These-2020-SML-Ecologie marine-BUREL Thomas.pdf.

I. Rezzoug & A. Ayadi, “Identification of an unknown part of the boundary of a Navier-stokes system by punctual sentinel”, Sciences & Technologie, 0 (2022), 31. https://www.asjp.cerist.dz/en/downArticle/406/0/ 35/61453.

G. Maykut & N. Untersteiner, “Some results from a time-dependent thermodynamic model of sea ice”, Journal of Geophysical Research, 76 (1971), 1550. https://doi.org/10.1029/JC076i006p01550.

B. Petrus, J. Bentsman & B. G. Thomas, Enthalpy-based feedback control algorithms for the Stefan problem, Proceedings of the 51st IEEE Conference on Decision and Control (CDC), Maui, HI, USA, 2012, pp. 7037– 7042. https://doi.org/10.1109/CDC.2012.6426035.

F. Conrad, D. Hilhorst & T. I. Seidman, “Well-posedness of a moving boundary problem arising in a dissolution-growth process”, Nonlinear Analysis: Theory, Methods & Applications, 15 (1990), 445. https: //doi.org/10.1016/0362-546X(90)90126-2.

B. Zalba, J. M. Marın, L. F. Cabeza & H. Mehling, “Review on thermal energy storage with phase change: materials, heat transfer analysis, and applications”, Applied Thermal Engineering, 23 (2003), 251. https://doi.org/10.1016/S1359-4311(02)00192-8.

V. Srinivasan & J. Newman, “Discharge model for the lithium-ironphosphate electrode”, Journal of the Electrochemical Society, 151 (2004), A1517. https://doi.org/10.1149/1.1785012.

F. Avner & F. Reitich, “Analysis of a mathematical model for the growth of tumors”, Journal of Mathematical Biology, 38 (1999), 262. https://doi. org/10.1007/s002850050149.

F. Wang, H. Wang, K. Xu, J. Wu & X. Jia, Characterizing information diffusion in online social networks with linear diffusive model, Proceedings of the 33rd International Conference on Distributed Computing Systems, Philadelphia, PA, USA, 2013, pp. 307–316. https://doi.org/10.1109/ ICDCS.2013.14.

O. Bodart & P. Demeestere, “Sentinels for the identification of an unknown boundary”, Mathematical Models and Methods in Applied Sciences, 07 (1997), 871. https://doi.org/10.1142/S021820259700044X.

C. Jose & G. Gianni, “La vitesse de propagation dans le probleme de la` digue”, Annales de la Faculte des sciences de Toulouse: Math´ ematiques,´ 11 (1990) 7. http://www.numdam.org/item/AFST1990 511370.pdf.

A. El Badia & F. Moutazaim, “A one-phase inverse Stefan problem”, Inverse Problems 15 (1999) 1507. https://doi.org/10.1088/0266-5611/15/6/ 308.

R. Demarque & E. Fernandez-Cara, “Local null controllability of one-´ phase Stefan problems in 2d star-shaped domains”, Journal of Evolution Equations 18 (2018) 245. https://doi.org/10.1007/s00028-017-0399-x.

S. Koga, M. Diagne & M. Krstic, “Control and state estimation of the one-phase Stefan problem via backstepping design”, IEEE Transactions on Automatic Control, 64 (2019) 510. https://doi.org/10.1109/TAC.2018.2836018.

J. L. Lions, “Exact controllability, stabilization, and perturbations for distributed systems”, SIAM Review, 30 (1988) 1. https://www.jstor.org/ stable/2031306.

J. Simon, “Domain variation for drag in stokes flow”, in Control Theory of Distributed Parameter Systems and Applications, X. Li & J. Yong (Ed.), Springer, Berlin, Heidelberg, 1991, pp. 28–42. https://doi.org/10.1007/ BFb0004434.

I. Rezzoug & T. E. Oussaief, “Approximate controllability”, WSEAS Transactions on Systems 19 (2020) 14. https://doi.org/10.37394/23202. 2020.19.3.

W. Shatanawi, T. Qawasmeh, A. Bataihah & A. Tallafha, “New contractions and some fixed point results with application based on extended quasi b-metric space”, UPB Scientific Bulletin, Series A: Applied Mathematics and Physics, 83 (2021) 39. https://www.scientificbulletin.upb.ro/ revdocsarhiva/fulle39 659665.pdf.

A. Bataihah, T. Qawasmeh & M. Shatnawi, “Discussion on b-metric spaces and related results in metric and G-metric spaces”, Nonlinear Functional Analysis and Applications 27 (2022) 233. https://doi.org/10.22771/nfaa.2022.2.7.02.02.

T. Qawasmeh & R. Hatamleh, “A new contraction based on H-simulation functions in the frame of extended b-metric spaces and application”, International Journal of Electrical and Computer Engineering 13 (2023) 4212. http://doi.org/10.11591/ijece.v13i4.pp4212-4221.

Published

2023-11-08

How to Cite

On sentinel method of one-phase Stefan problem. (2023). Journal of the Nigerian Society of Physical Sciences, 5(4), 1772. https://doi.org/10.46481/jnsps.2023.1772

Issue

Volume 5, Issue 4, November 2023 (In Progress)

Section

Original Research
Copyright & Licensing

Copyright (c) 2023 Merabti Nesrine Lamya, Iqbal M. Batiha, Imad Rezzoug, Adel Ouannas, Taki-Eddine Ouassaeif

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The Journal of the Nigerian Society of Physical Sciences (JNSPS) is published under the Creative Commons Attribution-NonCommercial 4.0 International (CC BY-NC 4.0)4.0 (CC BY-NC) license. This license was developed to facilitate open access, namely, it allows articles to be freely downloaded and to be re-used and re-distributed without restriction, as long as the original work is correctly cited. More specifically, anyone may copy, distribute or reuse these articles, create extracts, abstracts, and other revised versions, adaptations or derivative works of or from an article, mine the article even for commercial purposes, as long as they credit the author(s).

How to Cite

On sentinel method of one-phase Stefan problem. (2023). Journal of the Nigerian Society of Physical Sciences, 5(4), 1772. https://doi.org/10.46481/jnsps.2023.1772