Optimal control with the effects of ivermectin and live stock availability on malaria transmission

Abstract

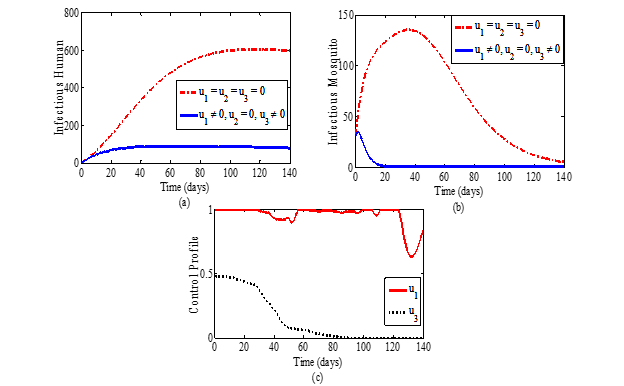
Malaria remains a global threat and the conventional methods used for combating the disease leave out mosquitoes that feed outdoors. This study addresses the challenge posed by such mosquitoes based on a tool called ivermectin drug which is lethal to mosquitoes that ingest bloodmeal containing a concentration of it. We formulated a mathematical model with three control tools (insecticide treated nets, treatment of infective individuals and ivermectin drug on livestock and humans) for the transmission and control of malaria under optimal condition. The model’s basic reproduction number, R₀ was estimated and the local and global stability analyses of the disease-free and endemic equilibrium points of the model were carried out. Sensitivity analysis carried out showed that R₀ is most sensitive to the mosquito biting rate and to the proportion of blood meal on human with cattle availability in such a way that any percent increase in the value of any of these parameters will lead to an equal percent increase in the value of R₀. The result of an optimal control analysis based on three time dependent controls suggests that the combination of all three controls gives the best result followed by the strategy that combines the use of ivermectin drug and the treatment of infective human. Depending on available resources, any of these is recommended to be adopted in malaria intervention programmes because of their effectiveness on both the infective human and mosquito populations with the potential of contributing significantly to the disease elimination within a minimal time frame.