Eigensolution to Morse potential for Scandium and Nitrogen monoiodides

Authors

  • C. A Onate Department of Physical Sciences, Landmark University, Omu-Aran, Nigeria.
  • G. O Egharevba Department of Physical Sciences, Landmark University, Omu-Aran, Nigeria.
  • D. T Bankole Department of Physical Sciences, Landmark University, Omu-Aran, Nigeria.

Keywords:

Eigensolutions, Wave equation, Bound state

Abstract

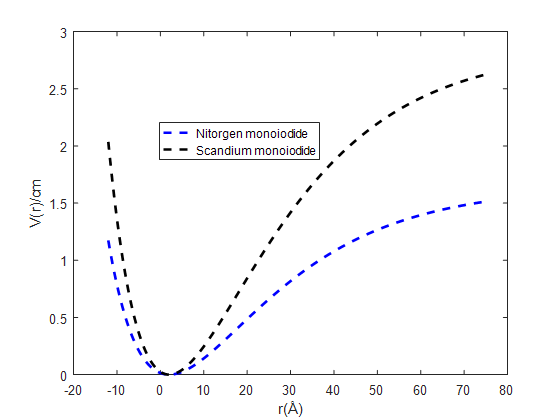
The solutions for Morse potential energy function under the influence of Schr¨odinger equation are examined using supersymmetric approach. The energy equation obtained was used to generate eigenvalues forX1 +state of scandium monoiodide (ScI) and X3 state of nitrogen monoiodide (NI) respectively were obtained by imputing their respective spectroscopic parameters. The calculated results for the two molecules aligned excellently with the predicted/observed values. 

Dimensions

O. Bayrak, I. Boztosun & H. Ciftci, “Exact analytical solutions of the Kratzer potential by the asymptotic iteration method”, International Journal of Quantum Chemistry 107 (2007) 540.

S. M. Ikhdair, “On the bound state solutions of the Manning-Rosen potential including an improved approximation to the orbital centrifugal term”, Physica Scripta 83 (2011) 10.

S. M. Ikhdair & R. Sever, “Exact quantization rule of the Kratzer-type potentials: An application to the diatomic molecules”, Journal of Mathematical Chemistry 45 (2009) 1137.

B. J. Falaye, K. J. Oyewumi, S. M. Ikhdair & M. Hamzavi, “Eigensolution techniques, their applications and Fisher’s information entropy of the Tietz-Wei diatomic molecular model”, Physica Scripta 89 (2014) 115204.

K. J. Oyewumi & K. D. Sen, “Exact solutions of the Schr?dinger equation for the pseudoharmonic potential: an application to some diatomic molecules”, Journal of Mathematical Chemistry 50 (2012) 1039.

C. A. Onate, A. Abolarinwa, S. O. Salawu & N. K. Oladejo, “Bound state solutions of the Schr?dinger equation and its application to some diatomic molecules”, Journal of Molecular Modeling 26 (2020) 145.

M. Farout, A. Bassalat & S. M. Ikhdair, “Exact quantizated momentum eigenvalues and eigenstates of a general potential model”, Journal of Applied Mathematical Physics 8 (2020) 1434.

R. Horchani, H. Jelassil, A. N. Ikot & U. S. Okorie, “Rotation vibration spectrum of potassium molecules via the improved generalized Pöschl-Teller oscillator”, International Journal of Quantum Chemistry 2020; e26558.

S. Kaur & C. G. Mahajan, “Some new four-parameter potentials and their use in the study of vibrational thermodynamic quantities of diatomic molecules”, Pramana Journal of Physics 52 (1999) 459.

J. Y. Liu, X.-T. Hu & C.-S. Jia, “Molecular energies of the improved Rosen?Morse potential energy model”, Canadian Journal of Chemistry 95 (2014) 40.

C. A. Onate & T. A. Akanbi, “Solutions of the Schrodinger equation with improved Rosen Morse potential for nitrogen molecule and sodium dimer”, Results in Physics 22 (2021) 103961

C. A. Onate, T. A. Akanbi & I. B. Okon, “Ro-vibrational energies of cesium dimer and lithium dimer with molecular attractive potential”, Scientific Reports 11 (2021) 6198.

C. A. Onate, M. C. Onyeaju, E. Omugbe, I. B. Okon & O. E. Osafile, “Bound-state solutions and thermal properties of the modified Tietz–Hua potential”, Scientific Reports 11 (2021) 2129.

X. T. Hu, J.-Y. Liu & C.-S. Jia, “The 33 +gstate of Cs2molecule”, Computational and Theoretical Chemistry 1019 (2013) 137.

M. L. da Silva, V. Guerra, L. Loureiro & P. A. Sa, “Vibrational distributions in N2 with an improved calculation of energy levels using the RKR method”, Chemical Physics 348 (2008) 187.

R. Horchani, N. Al-Kinda & H. Jelassi, “Ro-vibrational energies of caesium molecules with the Tietz-Hua oscillator”, Molecular Physics (2020) e1812746.

D. A. Morales, “Supersymmetric improvement of the Pekeris approximation for the rotating Morse potential”, Chemical Physics Letters 394 (2004) 68.

T. A. Akanbi, C. A. Onate & O. Y. Oludoun, “Eigensolutions and thermodynamicproperties of deformed and modifiedMorsepotential model”, AIP Advances 11 (2021) 045213.

M.Zarezadeh &M.K.Tavassoly, “The Solution of the Schrodinger equation for a particular form of Morse Potential by using Laplace Transform”, CPC(HEP &NP)33(2009) 1.

A.M.Desai, N.Mesquita&V.Fernandes, “AnewmodifiedMorsepotential energy function for diatomic molecules”, Physica Scripta 95 (2020) 085401

E. Witten, “Dynamical Breaking of Supersymmetry”, Nuclear Physics B 188 (1981) 513.

H. Hassanabadi, E. Maghsoodi, S. Zarrinkamar & H. Rahimov, “An Approximate solutions of the Dirac Equation for Hyperbolic scalar and vector potentials and a Coulomb tensor interaction by SUSY QM”, Modern Physics Letters A 26 (2011) 2703.

F. Cooper, A. Khare & U. Sukhatme, “Supersymmetry in Quantum Mechanics”, Physical Reports 251 (1995) 267.

C. A. Onate, “Relativistic and non-relativistic solutions of the inversely quadratic Yukawa Potential”, African Review of Physics 8 (2013) 325.

C. Tezcan & R. Sever, “A general approach for the exact Solution of the Schr¨odinger equation”, International Journal of Theoretical Physics 48 (2009) 337.

C. A. Onate, M. C. Onyeaju, A. N. Ikot, J. O. A. Idiodi & J. O. Ojonubah, “Eigen Solutions, Shannon Entropy and Fisher Information under the Eckart Manning Rosen Potential Model”, Journal of the Korean Physical Society 70 (2017) 339

R. R. Reddy, Y. N. Ahammed, B. S. Devi, P. A. Azeem, K. R. Gopal & T. V. R. Rao, “Potential energy curves, dissociation energies and Franck Condon factors for NI and ScI molecules”, Journal of Quantum Spectrosc andopy Radiation Transfer 74 (2002) 125

Published

2021-11-29

How to Cite

Eigensolution to Morse potential for Scandium and Nitrogen monoiodides. (2021). Journal of the Nigerian Society of Physical Sciences, 3(4), 282-286. https://doi.org/10.46481/jnsps.2021.407

Issue

Volume 3, Issue 4, November 2021

Section

Original Research
Copyright & Licensing

Copyright (c) 2021 Journal of the Nigerian Society of Physical Sciences

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Eigensolution to Morse potential for Scandium and Nitrogen monoiodides. (2021). Journal of the Nigerian Society of Physical Sciences, 3(4), 282-286. https://doi.org/10.46481/jnsps.2021.407