Characterisation of Singular Domains in Threshold-Dependent Biological Networks

Authors

  • Benitho Ngwu Department of Mathematics, Federal University Oye-Ekiti, Oye/Ekiti, Nigeria
  • Godwin C. E. Mbah Department of Mathematics, University of Nigeria, Nsukka/Enugu, Nigeria
  • Chika O. Mmaduakor Department of Mathematics, Federal University Oye-Ekiti, Oye/Ekiti, Nigeria
  • Sunday Isienyi Department of Mathematics & Statistics,Akanu Ibiam Federal Polytechnic Unwana, Afikpo/Ebonyi, Nigeria
  • Oghenekevwe R. Ajewole Department of Mathematics, Federal University Oye-Ekiti, Oye/Ekiti, Nigeria
  • Felix D. Ajibade Department of Mathematics, Federal University Oye-Ekiti, Oye/Ekiti, Nigeria

Keywords:

Discontinuous System, Singular Domain, Threshold-Dependent Network, Biological System, Piecewise Linear Models

Abstract

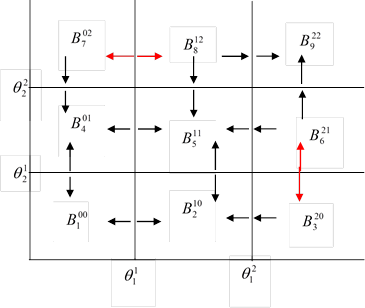
Threshold-dependent networks behave like piecewise smooth system which belongs to a class of systems with discontinuous right hand side. It can be studied with piecewise linear differential equations. At threshold values and their intersections, known as switching boundaries and surfaces, the state of such networks is not defined because of singularity at such points. These states are termed singular states or domains of the network. This study is to characterise, in terms of number, singular domains of any order in a network and the total number of such domains. It shall also propose new definitions for walls which are switching domains of order one. The new definition, which derives from Fillipov’s First Order Theory on characterisation of (sliding) wall, shall be shown to prove better than existing ones as it can reveal intrinsic behaviours in such networks.

Dimensions

K. H. Johansson, A. E. Barabanov & K. J. Astrom,” Limit cycles with chattering in relay feedback systems”. IEEE Trans. Automatic Contro 247 (2002) 1414.

M. Biak, T. Hanus & D. Janovska , "Some applications of Filippov’s dynamical systems”, Journal of Computational and Applied Mathematics 224 (2013) 132

V. I. Utkin, ”Variable structure systems with sliding modes”, IEEE Trans. Automatic Control 22 (1977) 212

E. K. P. Chong, S. Hui & S. H. Zak, ``An analysis of a class of neural networks for solving linear programmingproblems”, IEEE Trans. Autom. Control 44 (1999) 1995

R. Casey, H. de Jong, & J-L. Gouze, ``Piecewise-linear models of genetics regulatory networks: Equilibria and their stability”, J. Math. Biol., 52 (2006) 27

H. de Jong, J- L. Gouze, C. Hernandez, M. Page, T. Sari, & J. Geiselmann, ``Qualitative simulation of genetic regulatory networks using piecewiselinear models”, Bulletin of Mathematical Biology, 66 (2004) 301

K. al-Shammari, ``Filippov’s operator and discontinuous differential equations”, A Ph.D Dissertation submitted to the Graduate Faculty of the Louisiana State University and Agricultural and Mechanical College, USA. (2006) 31

F. Grognard, H. de Jong & J-L. Gouze, ``Piecewise-linear models of genetic regulatory networks: theory and example”, Isabelle Queinnec, Sophie Tarbouriech, Germain Garcia, SilviuIulianNiculescu. Biology and Control Theory: Current Challenges, 357, Springer, pp.137 - 159, Lecture Notes in Control and Information Sciences

A. Machina & A. Ponosov, ``Filippov solutions in the analysis of piecewise linear models describing gene regulatory networks", Nonlinear Anal. 74 (2011) 882

P. T. Piiroinen & Yu. A. Kuznetsov, ``An event-driven method to simulate Filippov systems with accurate computing of sliding motions”, ACM TOMS 34 (2008) 24

Y. A. Kuznetsov, S. Rinaldi & A. Grnani, ``One-parameter bi-furcations in planar Filippov systems”, International Journal of Bifuraction & Chaos 13 (2003) 2157

L. Dieci & L. Lopez, ”Sliding Motion in Filippov Differential Systems: Theoretical Results and a Computational Approach”, SIAM Journal on Numerical Analysis 47 (2009) 2023.

http://www.jstor.org/stable/27862720

J- L. Gouze & T. Sari, ”A class of piecewise linear differential equations arising in biological models”, Dynamical Systems 17 (2002) 303

G. Yagil & E. Yagil, ”On the relation between effector concentration and the rate of induced enzyme synthesis”, Journal of Biophysiology 11 (1971) 11

D. Ropers, H. Jong, M. Page, D. Schneider & J. Geiselmann, ”Qualitative simulation of the carbon starvation response in Escherichia coli”.

BioSystems 84 (2006) 124

H. de Jong, J- L. Gouz, C. Hernandez, M. Page, T. Sari & J. Geiselmann, ”Qualitative simulation of genetic regulatory networks using piecewise-linear models”. Bulletin of Mathematical Biology 66 (2004)

doi: 10.1016/j.bulm.2003.08.010. PMID: 14871568

T. Mestl, E Plahte & S. W. Omholt, ”Mathematical Framework for Describing and Analysing Gene Regulatory Networks”, Journal of Theoritical Biology 176 (1995) 292

D. D Noveas & M. A. Teixeira, ”Shilnikov problem in Filippov dynamical systems”, Chaos 29 (2019) 063110

Z. Du & Y. Li, ”Bifurcation of periodic orbits with multiple crossings in a class of planar Filippov systems”, Mathematical and Computer Modelling” 55 (2012) 1072

T. Li & X. Chen, ”Periodic orbits of linear Filippov systems with a line of discontinuity”, Qualitative Theory of Dynamical Systems 19 (2020) 1

P. R. da Silva & J. R. De Moras,” Piecewise Smooth Slow-Fast Systems”, Journal of Dynamical and Control Systems 27 (1) (2021) 1.

L. Dieci & C. Elia, ”Piecewise Smooth Systems near a Co-dimension Discontinuity Manifold: can one say what should happen”, Discrete Continuous Dynamical Systems 9(4) (1978) 1039.

L. Dieci, C. Elia & Z. Lopez, ”On Filippov Solutions of Discontinuous DAEs of index 1 ”, Communications in Nonlinear Science and Numerical Simulation 95 (2021)105656.

P. R. da Silva, I. S. Meza-Sarmiento & D. D. Noveas, ”Nonlinear Sliding Discontinuous Vector Field and Singular Perturbation”, Differential Equations and Dynamical Systems 30 (2018) 675.

E. Plahte, T. Mestl & S. W. Omholt, ”Global analysis of steady points for systems of differential equations with sigmoid interactions”, Dynamics and Stability of Systems: An International Journal 9 (1994) 7

A. Machina, R. Edwards, & P. van den Driessche, ``Singular Dynamics in Gene Network Models”, SIAM Journal of Applied DYnamical Systems 12 (2013) 96

F. Grognard, H. de Jong & J- L. Gouze, ``Piecewise-Linear Models of Genetic Regulatory Networks: Theory and Example”. In: Queinnec, I., Tarbouriech, S., Garcia, G., Niculescu, SI. (eds) Biology and Control Theory: Current Challenges. Lecture Notes in Control and Information Sciences, Springer, Berlin, Heidelberg. 357 (2007) 149.

R. Porreca, S. Drulhe H. de Jong & G. Ferrari-Trecate, ``Structural Identification of Piecewise-Linear Models of Genetic Regulatory Networks", Journal of Computational Biology 15 (2008) 8

L. Glass & J. S. Pasternack, ``Stable oscillations in mathematical models of biological control systems”, Journal of Mathematical Bioogy 6 (1978) 211.

E. H. Snoussi & R. Thomas, ``Logical identification of all steady states: the concept offeedback loop characteristic states", Bulletin of Mathematical Biology 5 (1993) 973

R. Casey, H. de Jong & J- L. Gouze, ``Piecewise-linear models of genetics regulatory net works: Equilibria and their stability", Journal of Mathematical Biology 52 (2006) 27.

E. Plahte & S. Kjoglum, Analysis and generic properties of gene regulatory networks with graded response functions”, Physica D 201 (2005) 155

Published

2022-08-20

How to Cite

Characterisation of Singular Domains in Threshold-Dependent Biological Networks. (2022). Journal of the Nigerian Society of Physical Sciences, 4(3), 812. https://doi.org/10.46481/jnsps.2022.812

Issue

Volume 4, Issue 3, August 2022

Section

Original Research
Copyright & Licensing

Copyright (c) 2022 Benitho Ngwu, Godwin C. E. Mbah, Chika O. Mmaduakor, Sunday Isienyi, Oghenekevwe R. Ajewole, Felix D. Ajibade

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Characterisation of Singular Domains in Threshold-Dependent Biological Networks. (2022). Journal of the Nigerian Society of Physical Sciences, 4(3), 812. https://doi.org/10.46481/jnsps.2022.812