On Nonexpansive and Expansive Semigroup of Order-Preserving Total Mappings in Waist Metric Spaces

Authors

  • O. T. Wahab Department of Mathematics and Statistics, Kwara State University, Malete, Nigeria https://orcid.org/0000-0002-5732-3930
  • I. F. Usamot Department of Mathematics, University of Ilorin, Ilorin, Nigeria
  • S. M. Alata Department of Computer Science, College of Arabic and Islamic Legal Studies, Ilorin, Nigeria
  • K. R. Tijani Department of Mathematical Sciences, Osun State University, Osogbo, Nigeria

Keywords:

Fixed point, semitopological semigroup, order-preserving total mappings, waist metric space, nonexpansive map

Abstract

In this paper, we introduce nonexpansive and expansive semigroup of order-preserving total mappings (ONTn) and (OETn), respectively, to prove some fixed point theorems in waist metric spaces. We examine the existence of mappings that satisfy the conditions ONTn and OETn. We also prove that every semigroup of order-preserving total mappings OTn has fixed point properties and that the set of fixed points is closed and convex. The present study generalised many previous results on semigroup of order-preserving total mappings OTn. Efficacy of the results was justified with some practical examples.

Dimensions

G. U. Garba, “Nilpotents in semigroups of partial one-to-one orderpreserving mappings”, Semigroup Forum 41 (1991) 1.

P. M. Higgins, Techniques of Semigroup Theory, Oxford University Press (1992).

P. M. Higgins, “Combinatorial results for semigroups of order-preserving mappings”, Math. Proc. Camb. Phil. Soc. 113 (1993) 281.

J. M. Howie, Fundamentals of semigroup theory, Oxford University Press Inc. New York (1995).

A. Umar, “Some combinatorial problems in the theory of symmetric inverse semigroups”, Algebra Discrete Math. 9 (2010) 115.

R. D. Holmes, A. T. Lau, “Nonexpansive actions of topological semigroups and fixed points”, J. Lond. Math. Soc. 5 (1972) 330.

H. S. Kim, T. H. Kim, “Asymptotic behavior of semigroups of asymptotically nonexpansive type on Banach spaces”, J. Korean Math. Soc. 24 (1987) 169.

A. T. Lau, Y. Zhang, “Fixed point properties of semigroups of nonexpansive mappings”, J. Funct. Anal. 254 (2008) 2534.

W. Phuengrattana, S. Suantai, “Fixed point theorems for a semigroup of generalized asymptotically nonexpansive mappings in CAT(0) spaces”, Fixed Point Theory and Applications 2012 (2012) 230. DOI: 10.1186/1687-1812-2012-230.

A. H. Soliman, “A tripled fixed point theorem for semigroups of Lipschitzian mappings on metric spaces with uniform normal structure”, Fixed point theory and Appl. 346 (2013) 1.

W. Takahashi, P.J. Zhang, “Asymptotic behavior of almost-orbits of semigroups of Lipschitzian mappings”, J. Math. Anal. Appl. 142 (1989) 242.

M. T. Kiang, “Fixed point theorems for certain classes of semigroups of mappings”, Transactions of the American Mathematical Society 189 (1974) 63.

J. C. Yao, L. C. Zeng, “Fixed point theorem for asymtotically regular semigroups in metric spaces with uniformly normal structure”, J. Nonlinear Convex Anal. 8 (2007) 153.

G. Ayik, H. Ayik, M. Koc, “Combinatorial results for order-preserving and order-decreasing transformations”, Turk J. Math. 35 (2011) 617.

A. Laradji, “Fixed points of order-preserving transformations”, Journal of Algebra and Its Applications, 21 (2022) 2250082.

S. Banach, “Surles Operations dans les eusembles abstraits et leur application aus equations integrales”, Fund. Math. 3 (1922) 133.

L. P. Belluce, W. A. Kirk, “Fixed point theorem for families of contraction mappings”, Pac. J. Math. 18 (1966) 213.

L. P. Belluce, W. A. Kirk, “Nonexpansive mappings and fixed point in Banach spaces”, III. J. Math. 11 (1967) 474.

F. E. Browder, “Nonexpansive of nonlinear operators in a Banach space”, Proc. Natl. Sci. USA. 54 (1965) 1041.

T. C. Lim, “A fixed point theorem for families of non-expansive mappings”, Pac. J. Math. 53 (1974) 487.

Y. Ibrahim, “Strong convergence theorems for split common fixed point problem of Bregman generalized asymptotically nonexpansive mappings in Banach spaces”, J. Nig. Soc. Phy. Sci. 1(2) (2019) 35

R. Hopkins, Finite metric spaces and their embedding into Lebesgue spaces, University of Chicago Mathematics REU (2015).

M. D. Guay, K. L. Singh, J. H. M. White, “Fixed point theorems for non-expansive mappings in convex metric spaces”, Proc. Conference on Nonlinear Analysis (Ed. S. P. Singh and J. H. Bury) Marcel Dekker 80 (1982) 179.

W. A. Takahashi, “A convex in metric space and non-expansive mappings I”, Kodai Math. Sem. Rep. 22 (1970) 142.

C. E. Chidume, Geometric Properties of Banach Spaces and Nonlinear Iterations, Verlag Series: Springer (2009).

F. Bruhat, J. Tits, “Groupes r’eductifs sur un corps local. I. Donn’ees radicielles valu’ees”, Inst. Hautes´E tudes Sci. Publ. Math. 41 (1972) 5.

A. Laradji & A. Umar, “Combinatorial results for semigroups of orderpreserving full transformations”, Semigroup Forum 72 (2006) 51

Springer-Verlag.

A. Laradji, A. Umar, “Combinatorial results for semigroups of orderpreserving partial transformations”, Journal of Algebra, 278 (2004), 342.

A. Laradji, A. Umar, “On certain finite semigroups of order-decreasing transformations I”, Semigroup Forum 69 (2004) 184.

I. Dimitrova, J. Koppitz, “On relative ranks of the semigroup of orientation-preserving transformations on infinite chains”, AsianEuropean Journal of Mathematics, 14 (2021) 2150146.

R. Tanyawong, R. Srithus, R. Chinram, “Regular subsemigroups of the semigroups of transformations preserving a fence”, Asian-European Journal of Mathematics 9 (2016) 1650003.

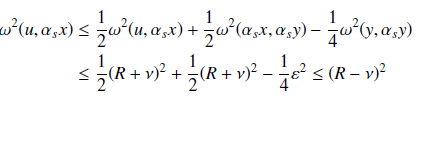
Published

2022-12-22

How to Cite

On Nonexpansive and Expansive Semigroup of Order-Preserving Total Mappings in Waist Metric Spaces. (2022). Journal of the Nigerian Society of Physical Sciences, 5(1), 878. https://doi.org/10.46481/jnsps.2023.878

Issue

Volume 5, Issue 1, February 2023

Section

Original Research
Copyright & Licensing

Copyright (c) 2022 O. T. Wahab, I. F. Usamot, S. M. Alata, K. R. Tijani

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

On Nonexpansive and Expansive Semigroup of Order-Preserving Total Mappings in Waist Metric Spaces. (2022). Journal of the Nigerian Society of Physical Sciences, 5(1), 878. https://doi.org/10.46481/jnsps.2023.878