On Lemniscate of Bernoulli of q-Janowski type

Afis Saliu; Semiu Oladipupo Oladejo

doi:10.46481/jnsps.2022.961

Authors

Afis Saliu
[email protected]
Department of Mathematics, Gombe State University P.M.B 127, Tudun Wada, Gombe, Gombe State, Nigeria; Department of Mathematics, University of the Gambia, MDI Road, Kanifing P.O. Box 3530, Serrekunda, The Gambia
Semiu Oladipupo Oladejo Department of Mathematics, Gombe State University P.M.B 127, Tudun Wada, Gombe, Gombe State, Nigeria

Keywords:

Univalent functions, Schwarz functions, Lemniscate of Bernoulli, Subordination, Janowski Functions

Abstract

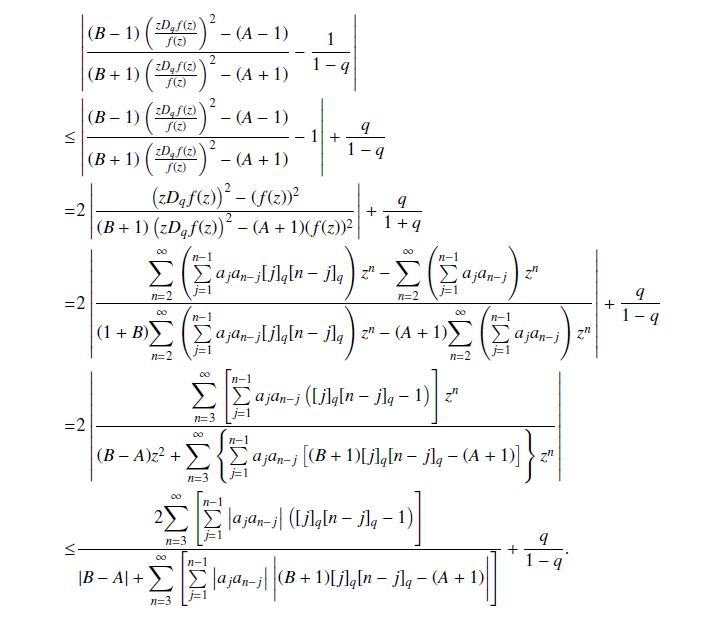
In this article, we introduce the q-analogue of functions characterized by the lemniscate of Bernoulli in the right-half plane and define the class $\mathbb{L}^{\ast}_{q}(A, B)$. Furthermore, we study the geometric properties of this class, which include coefficient inequalities, subordination factor sequence property, radii results and Fekete-Szeg$\ddot{\textup{o}}$ problems. Some deductions of our results show relevant connections between this present work and the existing ones in many literature. It is worthy of note that some of our results are sharp.

Dimensions

REFERENCES

A. W. Goodman, Univalent Functions, vols. I-II, United States of America, Mariner Publishing Company, Tempa. Florida (1983).

L. De Branges, “A proof of the Bieberbach conjecture”, Acta Math. 154 (1985) 137.

W. Ma & D. Minda, “A unified treatment of some special classes of univalent functions”: in Proceedings of the Conference on Complex Analysis, Tianjin, China, 19–23 June 1992; pp. 157–169.

J. Soko? & J. Stankiewicz, “Radius of convexity of some subclasses of´ strongly starlike functions”, Folia Scient. Univ. Tech. Res. 19 (1996) 101.

J. Soko?, “Coe´ ffcient estimates in a class of strongly starlike functions”, Kyungpook Math. J. 49 (2009) 349.

R. M. Ali, N. K. Jain & V. Ravichandran, “Radii of starlikeness associated with the lemniscate of Bernoulli and the left-half plane”, Appl. Math. Comput. 218 (2012) 6557.

J. Soko?, “Radius problems in the class´ SL”, Appl. Math. Comput. 214 (2009) 569.

M. Raza & S. N. Malik, “Upper bound of the third Hankel determinant for a class of analytic functions related with lemniscate of Bernoulli”, J. Inequal. Appl. 2013 (2013) 1.

A. Afis & K. I. Noor, “ On subclasses of functions with boundary and radius rotations associated with crescent domains”, Bull. Korean Math. Soc. 57 (2020) 1529.

B. Ahmad, M. Darus, N. Khan, R. Khan & M. G. Khan, “On a class of analytic multivalent analytic functions in q-analogue associated with leminiscate of Bernoulli”, Bull. Math. Anal. Appl. 13 (2021) 71.

S. Kanas, V. S. Masih, A. Ebadian, “Coefficients problems for families of holomorphic functions related to hyperbola”, Math. Slovaca 70 (2020) 605.

V. S. Masih & S. Kanas, “Subclasses of Starlike and Convex Functions Associated with the Limac¸on Domain”, Symmetry, 12 (2020) 942.

P. Sharma, R. K. Raina & J. Soko?, “Certain Ma-Minda type classes of an-´ alytic functions associated with the crescent-shaped region”, Anal. Math. Phys. 9 (2019) 1887-1903.

A. Saliu, “On generalized k-uniformly close-to-convex functions of Janowski type”, Int. J. Appl. Anal. 17 (2019) 958.

A. Saliu & K. I. Noor, “On Janowski close-to-convex functions associated with conic regions”, Int. J. Appl. Anal. 18 (2020) 614.

A. Saliu, K. I. Noor, S. Hussain & M. Darus, “On quantum differential subordination related with certain family of analytic functions”, J. Math. 2020 (2020) 6675732. https://doi.org/10.1155/2020/6675732.

A. Saliu, K. I. Noor, S. Hussain & M. Darus, “Some results for the family of univalent functions related with limac¸on domain”, AIMS Math. 6 (2021) 3410.

H. M. Srivastava, B. Tahir, B. Khan, Q. Z. Ahmad & N. Khan, “Some general classes of q-starlike functions associated with the Janowski functions”, Symmetry 11 (2019) 292.

H. E. O. Ucar, “Coe¨ fficient inequality for q-starlike functions”, Appl. Math. Comput. 276 (2016) 122.

M. Ul-Haq, M., Raza, M., Arif, Q. Khan & H. Tang, “q-Analogue of differential subordinations”, Mathematics 7 (2019) 724.

F. H. Jackson, “On q-functions and a certain difference operator”, Trans. Royal Soc. Edinburgh, 46 (1909) 253.

F. H. Jackson, “On q-definite integrals”, The Quarterly J. Pure Appl. Math. 41 (1910) 193.

M. E. H Ismail, E. Merkes & D. Styer, “ A generalization of starlike functions”, Complex Var. Elliptic Equ. 14 (1990) 77.

N. Khan, M. Shafiq, M., Darus, B. Khan & Q. Z. Ahmad, “Upper bound of the third Hankel determinant for a subclass of q-starlike functions associated with Lemniscate of Bernoulli”, J. Math. Inequal. 14 (2020) 51.

W. Janowski, “Some extremal problems for certain families of analytic functions I”, Ann. Polon. Math. 28 (1973) 297.

H. S. Wilf, “Subordinating factor sequences for convex maps of the unit circle”, Proc. Am. Math. Soc. 12 (1961) 689.

A. Aral, V. Gupta & R. P. Agarwal, Applications of q-calculus in Operator Theory, Springer. New York (2013) 262.

R. M. Ali, V. Ravichandran & N. Seenivasagan, “Coefficient bounds for p-valent functions”, Appl. Math. Comput. 187 (2007) 35.

K. I. Noor, S. Riaz & M. A. Noor, “On q-Bernardi integral operator”, TWMS J. Pure Appl. Math. 8 (2017) 3.

K. I. Noor & S. Riaz, “ Generalized q-starlike functions”, Stud. Sci. Math. Hung. 54 (2017) 509.

K. I. Noor, “Some classes of q-alpha starlike and related analytic functions”, J. Math. Anal. 8 (2017) 24.

S. E. Fadugba, “Solution of fractional order equations in the domain of the Mellin transform”, J. Nig. Soc. Phys. Sci. 1 (2019) 138. https://doi.org/10.46481/jnsps.2019.31

L. K. Alzaki, & H. K. Jassim, “Time-Fractional Differential Equations with an Approximate Solution”, J. Nig. Soc. Phys. Sci. 4 (2022) 818. https://doi.org/10.46481/jnsps.2022.818