Simple motion pursuit differential game problem of many players with integral and geometric constraints on controls function.
Keywords:
Differential game, pursuer, evader, geometric constraint, integral constraint, Hilbert spaceAbstract
We study a simple motion pursuit differential game of many pursuers and one evader in a Hilbert space $l_{2}$. The control functions of the pursuers and evader are subject to integral and geometric constraints respectively. Duration of the game is denoted by positive number $\theta $. Pursuit is said to be completed if there exist strategies $u_{j}$ of the pursuers $P_{j}$ such that for any admissible control $v(\cdot)$ of the evader $E$ the inequality $\|y(\tau)-x_{j}(\tau)\|\leq l_{j}$ is satisfied for some $ j\in \{1,2, \dots\}$ and some time $\tau$. In this paper, sufficient conditions for completion of pursuit were obtained. Consequently strategies of the pursuers that ensure completion of pursuit are constructed.
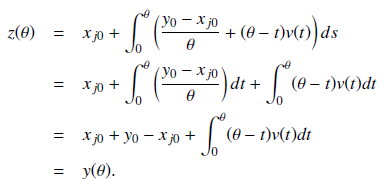
Published
How to Cite
Issue
Section
How to Cite
Most read articles by the same author(s)
- Jamilu Adamu, Aminu Sulaiman Halliru, Bala Ma’aji Abdulhamid, On Some Pursuit Differential Game Problem in a Hilbert Space , Journal of the Nigerian Society of Physical Sciences: Volume 4, Issue 1, February 2022







