Some theorems on fixed points in bi-complex valued metric spaces  with an application to integral equations

Authors

  • A. Murali School of Advanced Sciences, VIT University, Chennai-600 127, Tamil Nadu, India
  • K. Muthunagai School of Advanced Sciences, VIT University, Chennai-600 127, Tamil Nadu, India

Keywords:

common fixed point, metric space, control functions, Rational expressions

Abstract

Recent studies have highlighted fixed point theorems in the context of bicomplex valued metric spaces, utilizing rational type contractions with coefficients defined by two-variable control functions. In our research, we extend these findings by proposing new theorems for identifying common fixed points within bicomplex valued metric spaces, employing rational type contractions characterized by three-variable control functions as coefficients. We have refined the contraction conditions presented in numerous existing theorems by substituting constants with a limited number of control functions for more versatility in bicomplex valued metric spaces. This advancement broadens the scope of several significant findings in the literature. To demonstrate the efficacy of our results, we offer compelling examples that validate our theorems. Furthermore, we apply our primary findings to effectively address the Urysohn integral equation system, showcasing the practical application of our research.

Dimensions

W. A. Kirk, “Some recent results in metric fixed point theory”, J Fixed Point Theory Appl. 2 (2007) 195. https://doi.org/10.1007/s11784-007-0031-8

C. Semple, & M. Steel, Phylogenetics, Oxford Lecture Ser. In Math Appl, vol. 24, Oxford Univ. Press, Oxford, 2003.

A. I. Perov, “On the Cauchy problem for a system of ordinary difierential equations”, Pvi-blizhen Met Reshen Diff Uvavn. 2 (1964) 115-134.

Z. Mustafa, & B. Sims, “A new approach to generalized metric spaces”, J Nonlinear Convex Anal. 7 (2006) 289.

L. G. Huang & X. Zhang, “Cone metric spaces and fixed point theorems of contractive mappings”, J Math Anal Appl. 332 (2007) 1468. https://doi:10.1016/j.jmaa.2005.03.087.

V. V. Chistyakov,“Modular metric spaces I: basic concept”, Nonlinear Anal. 72 (2010) 1. https://doi:10.1016/j.na.2009.04.057.

Y. Ibrahim, “Common fixed point theorems for multivalued generalized F-Suzuki-contraction mappings in complete strong b-metric spaces”, Journal of the Nigerian Society of Physical Sciences 1 (2019) 88. https://doi.org/10.46481/jnsps.2019.19.

Y. Ibrahi, “Strong convergence theorems for split common fixed point problem of Bregman generalized asymptotically nonexpansive mappings in Banach spaces”, Journal of the Nigerian Society of Physical Sciences 1 (2019) 35. https://doi.org/10.46481/jnsps.2019.9

G. B. Price, “An introduction to multicomplex spaces and functions”, CRC Press 1991. https://doi.org/10.1201/9781315137278.

A. Azam, B. Fisher & M. Khan, “Common fixed point theorems in complex valued metric spaces”, Numer. Funct. Anal. Optim. 32 (2011) 243. https://doi.org/10.1080/01630563.2011.533046.

J. Ahmad, A. Azam & S. Saejung, “Common fixed point results for contractive mappings in complex valued metric spaces”, Fixed Point Theory and Applications 2014 (2014) 67 https://doi.org/10.1186/1687-1812-2014-67.

C. Klin-eam & C. Suanoom, “Some common fixed-point theorems for generalized-contractive-type mappings on complex-valued metric spaces”, Abstr. Appl. Anal. 2013 (2013) 604215. https://doi.org/10.1155/2013/604215.

F. Rouzkard & M. Imdad, “Some common fixed point theorems on complex valued metric spaces”, Comput. Math. Appl. 64 (2012) 1866. https://doi.org/10.1016/j.camwa.2012.02.063.

H. K. Nashine, Imdad & M. Hasan, “Common fixed point theorems under rational contractions in complex valued metric spaces”, J. Nonlinear Sci. Appl. 7 (2014) 42.

W. Sintunavarat & P. Kumam, “Generalized common fixed point theorems in complex valued metric spaces and applications”, J. Inequal. Appl. (2012) 1. https://doi.org/10.1186/1029-242X-2012-84.

W. Sintunavarat, Y. J. Cho, & P. Kumam, “Urysohn integral equations approach by common fixed points in complex valued metric spaces”, Adv. Differ. Equ. 1 (2013) 1. https://doi.org/10.1186/1687-1847-2013-49

K. Sitthikul, & S. Saejung, “Some fixed point theorems in complex valued metric spaces”, Fixed Point Theory Appl. 2012 (2012) 189. https://doi.org/10.1186/1687-1812-2012-189.

N. Singh, D. Singh, A. Badal , & V. Joshi, “Fixed point theorems in complex valued metric spaces”, Journal of the Egyptian Mathematical Society 24 (2016) 402. https://doi.org/10.1016/j.joems.2015.04.005.

J. Choi, S. K. Datta, T. Biswas & N. Islam, “Some fixed point theorems in connection with two weakly compatible mappings in bicomplex valued metric spaces”, Honam Math. J. 39 (2017) 115. https://doi.org/10.5831/HMJ.2017.39.1.115

I. H. Jebril, S. K. Datta, R. Sarkar & N. Biswas, “Common fixed point theorems under rational contractions for a pair of mappings in bicomplex valued metric spaces”, J. Interdiscip. Math. 22 (2019) 1071. https://doi.org/10.1080/09720502.2019.1709318

A. J. Gnanaprakasam, S. M. Boulaaras, G. Mani, B. Cherif & S. A. Idris, “Solving system of linear equations via bicomplex valued metric space”, Demonstr. Math. 54 (2021) 474. https://doi.org/10.1515/dema-2021-0046.

A.Tassaddiq, J.Ahmad, A.E. Al-Mazrooei, D. Lateef & F. Lakhani, “On common fixed point results in bicomplex valued metric spaces with application”, AIMS Mathematics 8 (2023) 5522.

I. Beg, S. K. Datta & D. Pal, “Fixed point in bicomplex valued metric spaces”, Int. J. Nonlinear Anal. Appl. 12(2021) 717. https://doi.org/10.22075/IJNAA.2019.19003.2049.

Z. Gu, G. Mani, A. J. Gnanaprakasam & Y. Li, “Solving a system of nonlinear integral equations via common fixed point theorems on bicomplex partial metric space”, Mathematics 9 (2021) 15. https://doi.org/10.3390/math9141584.

1750

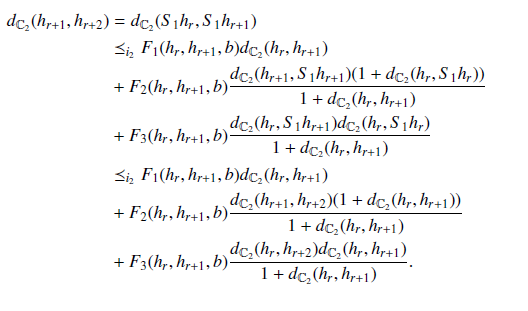
Published

2024-03-13

How to Cite

Some theorems on fixed points in bi-complex valued metric spaces  with an application to integral equations. (2024). Journal of the Nigerian Society of Physical Sciences, 6(2), 1750. https://doi.org/10.46481/jnsps.2024.1750

Issue

Volume 6, Issue 2, May 2024 (In Progress)

Section

Mathematics & Statistics
Copyright & Licensing

Copyright (c) 2024 A. Murali, K. Muthunagai

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Some theorems on fixed points in bi-complex valued metric spaces  with an application to integral equations. (2024). Journal of the Nigerian Society of Physical Sciences, 6(2), 1750. https://doi.org/10.46481/jnsps.2024.1750