Convolution equation and operators on the Euclidean motion group
Keywords:
Euclidean Motion group, invariant differential operator, Distribution, Universal enveloping algebraAbstract
Let $G = \mathbb{R}^2\rtimes SO(2)$ be the Euclidean motion group, let g be the Lie algebra of G and let U(g) be the universal enveloping algebra of g. Then U(g) is an infinite dimensional, linear associative and non-commutative algebra consisting of invariant differential operators on G. The Dirac measure on G is represented by $\delta_G$, while the convolution product of functions or measures on G is represented by $\ast$. Among other notable results, it is demonstrated that for each u in U(g), there is a distribution E on G such that the convolution equation $u\ast E = \delta_G$ is solved by method of convolution. Further more, it is established that the (convolution) operator $A^\prime : C^\infty_c(G)\rightarrow C^\infty(G),$, which is defined as $A^\prime f = f\ast T^n\delta(t)$ extends to a bounded linear operator on $L^2(G)$, for $f\in C^\infty_c(G)$, the space of infinitely differentiable functions on G with compact support. Furthermore, we demonstrate that the left convolution operator LT denoted as $L_Tf = T\ast f$ commutes with left translation, for $T\in D^\prime(G)$.
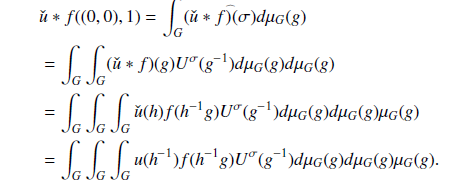
Published
How to Cite
Issue
Section
Copyright (c) 2024 U. N. Bassey, U. E. Edeke

This work is licensed under a Creative Commons Attribution 4.0 International License.







