On mathematical modelling of optimal control of typhoid fever with efficiency analysis
Keywords:
Typhoid fever, Medically hygienic, Optimal control, Efficiency analysisAbstract
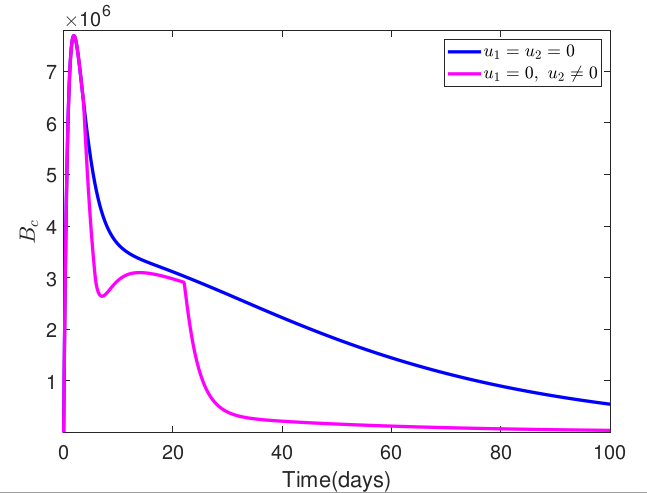
Typhoid fever is a fatal infectious disease that is endemic in most parts of the world, accounting for millions of cases and thousands of deaths from the disease annually. Despite several mathematical models for control of the disease, typhoid fever remains a threat, especially in Africa, South and Southeast Asia, South America and the Indian subcontinent, necessitating the need to propose an optimal control strategy for the transmission dynamics of typhoid fever. Thus, this research formulates an optimal control model for the transmission dynamics of typhoid fever by including a medically hygienic compartment in the model. To reduce the spread of the disease, the study incorporates environmental sanitation with personal hygiene practices and medical treatment as control strategies to examine their combined impact on typhoid fever prevention and control. The necessary conditions for the existence of the optimal solution to the formulated optimal control problem were derived based on Pontryagin's Maximum Principle. The resulting optimality system was then solved numerically using the fourth-order Runge-Kutta-based scheme. Also, the finding simulation demonstrated the effectiveness of the proposed control strategies in preventing the spread of the disease. In addition, an efficiency analysis was carried out to determine which combinations of the control strategies would be most effective in controlling the alarming spread of the disease. The findings from our study indicate that a combination of environmental sanitation with personal hygiene and treatment was the most efficient in controlling the spread of typhoid fever.
Published
How to Cite
Issue
Section
Copyright (c) 2024 Omowumi F. Lawal, Tunde T. Yusuf, Afeez Abidemi

This work is licensed under a Creative Commons Attribution 4.0 International License.
How to Cite
Most read articles by the same author(s)
- Tunde Tajudeen Yusuf, Afeez Abidemi, Ayodeji Sunday Afolabi, Emmanuel Jesuyon Dansu, Optimal Control of the Coronavirus Pandemic with Impacts of Implemented Control Measures , Journal of the Nigerian Society of Physical Sciences: Volume 4, Issue 1, February 2022







