Optimal Control of the Coronavirus Pandemic with Impacts of Implemented Control Measures

Authors

  • Tunde Tajudeen Yusuf Department of Mathematical Sciences, Federal University of Technology, Akure, P.M.B. 704, Ondo State, Nigeria
  • Afeez Abidemi Department of Mathematical Sciences, Federal University of Technology, Akure, P.M.B. 704, Ondo State, Nigeria https://orcid.org/0000-0003-1960-0658
  • Ayodeji Sunday Afolabi Department of Mathematical Sciences, Federal University of Technology, Akure, P.M.B. 704, Ondo State, Nigeria
  • Emmanuel Jesuyon Dansu Department of Mathematical Sciences, Federal University of Technology, Akure, P.M.B. 704, Ondo State, Nigeria

Keywords:

Coronavirus, Epidemiological model, Objective functional, Optimality system, Pontryagin’s maximum principle, Disease prevalence

Abstract

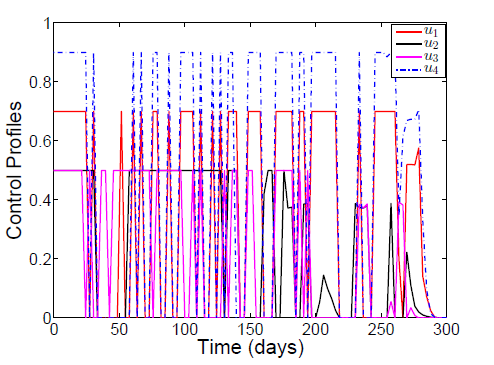
This paper considers the current global issue of containing the coronavirus pandemic as an optimal control problem. The goal is to determine the most advantageous levels of effectiveness of the various control and preventive measures that should be attained in order to cost effectively drive the epidemic towards eradication within a relatively short time. Thus, the problem objective functional is constructed such that it minimizes the prevalence as well as the cost of implementing the various control measures subject to a model for the disease transmission dynamics which incorporates the existing controls. The optimality system of the model is derived based on Pontryagin's maximum principle while the resulting system is solved numerically using the Runge-Kutta fourth order scheme with forward-backward sweep approach. Findings from our results show that the new cases and the prevalence of the disease can be remarkably reduced in a cost effective way, if the specified optimal levels of effectiveness of the various preventive and control measures are upheld continuously for at least a month. Moreover, the results also show that the disease can be eventually eradicated if these effectiveness levels are sustained over a reasonable length of time.

Dimensions

M. A. Shereen, S. Khan, A. Kazmi, N. Bashir, R. Siddique, COVID-19 infection: Origin, transmission, and characteristics of human coronaviruses, J. Adv. Res. 24 (2020) 91–98. doi:https://doi.org/10.1016/j.jare.2020.03.005.

R. Wu, L. Wang, H. C. D. Kuo, A. Shannar, R. Peter, P. J. Chou, S. Li, R. Hudlikar, X. Liu, Z. Liu, G. J. Poiani, L. Amorosa, L. Brunetti, A. N. Kong, An update on current therapeutic drugs treating COVID-19, Curr. Pharmacol. Rep. 6 (2020) 56–70. doi:https://doi.org/10.1007/s40495-020-00216-7.

F. Galluccio, T. Ergonenc, A. G. Martos, A. E. Allam, M. P´erez-Herrero, R. Aguilar, G. Emmi, M. Spinicci, I. T. Juan, M. Fajardo-P´erez, Treatment algorithm for COVID-19: A multidisciplinary point of view, Clin. Rheumatol. 39 (2020) 2077–2084. doi:https://doi.org/10.1007/270 s10067-020-05179-0.

R. C. Becker, COVID-19 treatment update: Follow the scientific evidence, J. Thromb. Thrombolysis 50 (2020) 43–53. doi:https://doi.org/10.1007/s11239-020-02120-9.

C. J. Galvin, Y. C. Li, S. Malwade, S. Syed-Abdul, COVID-19 preventive measures showing an unintended decline in infectious diseases in Taiwan, Int. J. Infect. Dis. 98 (2020) 18–20. doi:https://doi.org/10.1016/j.ijid.2020.06.062.

N. H. Shah, A. H. Suthar, E. N. Jayswal, Control strategies to curtail transmission of COVID-19, Int. J. Math. Math. Sci. 2020 (2020). doi:https://doi.org/10.1155/2020/2649514.

C. Tsay, F. Lejarza, M. A. Stadtherr, M. Baldea, Modeling, state estimation, and optimal control for the US COVID-19 outbreak, Sci. Rep. 10 (2020) 10711. doi:https://doi.org/10.1038/280 s41598-020-67459-8.

C. N. Ngonghala, E. Iboi, S. Eikenberry, M. Scotch, C. R. MacIntyre, M. H. Bonds, A. B. Gumel, Mathematical assessment of the impact of non-pharmaceutical interventions on curtailing the 2019 novel coronavirus, Math. Biosci. 325 (2020) 108364. doi:https://doi.org/10.1016/j.mbs.2020.108364.

[9] D. Okuonghae, A. Omame, Analysis of a mathematical model for COVID-19 population dynamics in Lagos, Nigeria, Chaos Solitons Fractals 139 (2020) 110032. doi:https://doi.org/10.1016/j.chaos.2020.110032.

World Health Organization, Coronavirus disease (COVID-2019) Situation Reports-193, Accessed on 9th August, 2020. URL https://www.who.int/docs/default-source/coronaviruse/situation-reports/20200731-covid-19-sitrep-193.pdf?sfvrsn=42a0221d_4

Nigeria Centre for Disease Control, COVID-19 outbreak in Nigeria situation report; NCDC: Abuja, Nigeria, Accessed on 8th August, 2020. URL https://ncdc.gov.ng/diseases/sitreps

C. Ohia, A. S. Bakarey, T. Ahmad, COVID-19 and Nigeria: Putting the realities in context, Int. J. Infect. Dis. 95 (2020) 279–281. doi:https://doi.org/10.1016/j.ijid.2020.04.062.

B. Ebenso, A. Otu, Can Nigeria contain the COVID-19 outbreak using lessons from recent epidemics?, Lancet Glob. Health 8 (6) (2020) e770. doi:https://doi.org/10.1016/S2214-109X(20)30101-7.

I. A. Osseni, COVID-19 pandemic in Sub-Saharan Africa: Preparedness, response, and hidden potentials, Trop. Med. Health 48 (2020) 48. doi:https://doi.org/10.1186/s41182-020-00240-9.

C. O. Ijalana, T. T. Yusuf, Optimal control strategy for Hepatitis B virus epidemic in areas of high endemicity, Int. J. Sci. Innov. Res. 5 (12) (2017) 28–39. doi:http://dx.doi.org/10.20431/2347-3142.0512003.

T. T. Yusuf, A. O. Olayinka, Optimal control of Meningococcal Meningitis transmission dynamics: A case study of nigeria, IOSR J. Math. 15 (3) (2019) 13–26.

L. L. Obsu, S. F. Balcha, Optimal control strategies for the transmission risk of COVID-19, J. Biol. Dyn. 1 (2020) 590–607. doi:https://doi.org/10.1080/17513758.2020.1788182.

A. Yousefpour, H. Jahanshahi, S. Bekiros, Optimal policies for control of the novel coronavirus disease (COVID-19) outbreak, Chaos Solitons Fractals 136 (2020) 109883. doi:https://doi.org/10.1016/j.chaos.2020.109883.

T. T. Yusuf, E. J. Dansu, A. Abidemi, A. S. Afolabi, Modelling the novel coronavirus (COVID-19) transmission dynamics with qualitative analysis: A case study of Nigeria, Submitted manuscript (2020).

A. Abidemi, R. Ahmad, N. A. B. Aziz, Global stability and optimal control of dengue with two coexisting virus serotypes, MATEMATIKA: Malaysian J. Ind. Appl. Math. 35 (4) (2019) 149–170. doi:https://doi.org/10.11113/matematika.v35.n4.1269.

A. Abidemi, N. A. B. Aziz, Optimal control strategies for dengue fever spread in Johor, Malaysia, Comput. Methods Programs Biomed. 196 (2020) 105585. doi:https://doi.org/10.1016/j.cmpb.2020.105585.

J. K. K. Asamoah, M. A. Owusu, Z. Jin, F. T. Oduro, A. Abidemi, E. O. Gyasi, Global stability and cost-effectiveness analysis of COVID-19 considering the impact of the environment: Using data from Ghana, Chaos Solitons Fractals 140 (2020) 110103. doi:https://doi.org/10.1016/j.chaos.2020.110103.

T. T. Yusuf, F. Benyah, Optimal control of vaccination and treatment for an SIR epidemiological model, World J. Model. Simul. 8 (3) (2012) 194–204.

T. T. Yusuf, F. Benyah, Optimal strategy for controlling the spread of HIV/AIDS disease: A case study of South Africa, J. Biol. Dyn. 6 (2) (2012) 475–494.

W. H. Fleming, R. W. Rishel, Deterministic and stochastic optimal control, Springer-Verlag, New York, 1975.

E. A. Coddington, N. Levinson, Theory of ordinary differential equations, McGraw Hill, New York, 1955.

L. S. Pontryagin, V. G. Boltyanskii, R. V. Gamkrelidze, E. F. Mishchenko, The mathematical theory of optimal processes, Gordon and Breach Science Publishers, 1986.

R. F. Hartl, Optimal control of non-linear advertising models with replenishable budget, Optim. Control Appl. Methods 3 (1) (1982) 53–65. doi:https://doi.org/10.1002/oca.4660030105.

S. Lenhart, J. T. Workman, Optimal control applied to biological models, Taylor & Francis, Boca Raton, FL, 2007.

United Nation, World population prospects, Accessed on 20th August, 2020. URL https://esa.un.org/unpd/wpp/DataQuery/

T. T. Yusuf, Mathematical modelling and simulation of Meningoccal Meningitis transmission dynamics, FUTA J. Res. Sci. 14 (1) (2018) 94–104.

Nigeria Centre for Disease Control, COVID-19 outbreak in Nigeria situation report; NCDC: Abuja, Nigeria, Accessed on 20 May, 2020. URL https://ncdc.gov.ng/diseases/sitreps

Published

2022-02-27

How to Cite

Optimal Control of the Coronavirus Pandemic with Impacts of Implemented Control Measures. (2022). Journal of the Nigerian Society of Physical Sciences, 4(1), 88-98. https://doi.org/10.46481/jnsps.2022.414

Issue

Volume 4, Issue 1, February 2022

Section

Original Research
Copyright & Licensing

Copyright (c) 2022 Journal of the Nigerian Society of Physical Sciences

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Optimal Control of the Coronavirus Pandemic with Impacts of Implemented Control Measures. (2022). Journal of the Nigerian Society of Physical Sciences, 4(1), 88-98. https://doi.org/10.46481/jnsps.2022.414