Unsteady Prandtl nanofluidic flow through stretching sheet due to mixed convection, first-order slip, and chemical reaction

Authors

  • V. Ramanjini Department of Mathematics, Government First Grade College Manvi, Raichur –584123, Karnataka, India
  • G. Gopi Krishna Department of Mathematics, Marri Laxman Reddy Institute of Technology and Management, Dundigal, Hyderabad – 500 043, India
  • Mani Ramanuja Department of Mathematics, Marri Laxman Reddy Institute of Technology and Management, Dundigal, Hyderabad – 500 043, India
  • Hari Kamala Sree Department of H & S, Malla Reddy College of Engineering & Technology - 500100, India
  • S. R. Mishra Department of Mathematics, Siksha ‘O’ AnusandhanDeeed to be University, Bhubaneswar - 751030, Odisha, India

Keywords:

Unsteady flow, Prandtlnanofluid, Thermal and solutal Buoyancy conditions, OHAM

Abstract

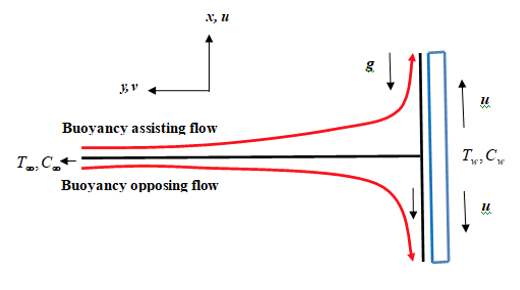
This article explores the unsteady flow, heat, and mass transport of a Prandtl nanofluid flow over a stretching sheet due to the existence of mixed convection, first-order slip, and chemical reaction. The flow characteristics are analysed for the interaction of thermal and solutal Biot numbers. The system of coupled non-linear leading partial differential equations (PDEs) is converted into non-linear ordinary differential equations (ODEs) with the facilitation of given similarity transformations. Furthermore, these equations are solved by applying a semi-analytical method termed the “Optimal Homotopy Analysis Method” (OHAM) using the computational software MATHEMATICA. The tables and Figures present the numerical computations of the pertinent parameters of the flow problems. The essential findings of this study are for the growing values of the Prandtl parameter, the velocity profile is enhanced, and the temperature profiles show the opposite behaviour. Unsteady parameters reduced the thickness of the heat and moving boundary layers. The temperature profiles are enhanced as the thermal Biot number grows, and similar characteristics can be observed in the solutal Biot number on concentration profiles.

Dimensions

B. C. Sakiadis, “Boundary layer behavior on a continuous solid surface: I. Boundary layer equations for two dimensional and axisymmetric flow”, Amer. Inst. Ch. Eng. J. 7 (1961) 26. https://doi.org/10.1002/aic.690070108

B. C. Sakiadis, “Boundary layer behavior on a continuous solid surface: II. The boundary layer on a continuous flat surface”, Amer. Inst. Ch. Eng. J. 7 (1961) 221. https://doi.org/10.1002/aic.690070211

F. K. Tsou, E. M. Sparrow & R. J. Goldstain, “Flow and heat transfer in the boundary on a continuous moving surface”, Int. J. Heat Mass Transf. 10 (1967) 219. https://doi.org/10.1016/0017-9310(67)90100-7

L. J. Crane, “Flow past a stretching plate”, Journal of Applied Mathematics and Physics (ZAMP) 21 (1970) 645. https://doi.org/10.1007/BF01587695

B. K. Dutta, P. Roy & A. S. Gupta, “Temperature field in flow over a stretching sheet with uniform heat flux”, Int. Commun. Heat Mass Trans. 12 (1985) 89. https://doi.org/10.1016/0735-1933(85)90010-7

L. J. Grubka & K. M. Bobba, “Heat transfer characteristics of a continuous stretching surface with variable temperature”, J. Heat Transfer. 107 (1985) 248. https://doi.org/10.1115/1.3247387

C. K. Chen & M. I. Char, “Heat transfer of a continuous stretching surface with suction and blowing”, ournal of Mathematical Analysis and Applications 135 (1988) 568. https://doi.org/10.1016/0022-247X(88)90172-2

M. Y. Malik, T. Salahuddin, A. Hussain & S. Bilal, “MHD flow of tangent hyperbolic fluid over a stretching cylinder: using Keller box method”, Journal of Magnetism and Magnetic Materials 395 (2015) 271. https://doi.org/10.1016/j.jmmm.2015.07.097

N. S. Akbar, A. Ebaid & Z. H. Khan, “Numerical analysis of magnetic field effects on Eyring-Powell fluid flow towards a stretching sheet”, Journal of Magnetism and Magnetic Materials 382 (2015) 355. https://doi.org/10.1016/j.jmmm.2015.01.088

S. U. S. Choi & J. A. Eastman, Enhancing thermal conductivity of fluids with nanoparticle, In D. A. Siginer and H. P. Wang (Eds.), Developments and Applications of Non-Newtonian Flows, ASME, New York, Vol. 66, 1995, pp. 99-105. https://ecotert.com/pdf/196525_From_unt-edu.pdf

C. H. Chon & K. D. Kihm, “Thermal conductivity enhancement of nanofluids by Brownian motion”, J. Heat Transfer. 127 (2005) 810. https://doi.org/10.1115/1.2033316

C. H. Li & G. P. Peterson, “Experimental investigation of temperature and volume fraction variations on the effective thermal conductivity of nanoparticle suspensions (nanofluids)”, J. Appl. Phys. 99 (2006) 084314. https://doi.org/10.1063/1.2191571

J. Buongiorno, “Convective transport in nanofluids”, J. Heat Transfer. 128 (2006) 240. https://doi.org/10.1115/1.2150834

B. Krishnendu, H. Tasawar & A. Ahmed, “Analytic solution for magneto hydrodynamic boundary layer flow Casson fluid over a stretching/shrinking sheet with wall mass transfer”, Chin. Phy. B. 22 (2013) 522. https://doi.org/10.1088/1674-1056/22/2/024702

K. V. Prasad, V. Hanumesh, K. Vajravelu & V. Ramanjini, “Analytical study of Cattaneo-Christov heat flux model for Williamson-nanofluid flow over a slender elastic sheet with variable thickness”, J. of Nonofluid. 7 (2018) 583. https://doi.org/10.1166/jon.2018.1475

I. Tlili & T. A. Alkanhal, “Nanotechnology for water purification: electrospun nanofibrous membrane in water and wastewater treatment”, J.Water Reuse and Desalination. 9 (2019) 232. https://doi.org/10.2166/wrd.2019.057

P. K. Dadheech, P. Agrawal & A. Sharma, K. S. Nisar & S. D. Purohit, “Marangoni convection flow of ?–AL2O3 nanofluids past a porous stretching surface with thermal radiation effect in the presence of an inclined magnetic field”, Heat Transfer 51 (2022) 534. https://doi.org/10.1002/htj.22318

R. E. Mfon, J. J. Deshi, Z. Al Amri & J. S. Madugu, “Biochemical synthesis, characterization and electrodeposition silver nanoparticles on a gold substrate”, J. Nig. Soc. Phys. Sci. 4 (2022) 796. https://doi.org/10.46481/jnsps.2022.796

I. Tlili, D. Baleanu, S. M. Sajadi, F. Ghaemi & M. A. Fagiry, “Numerical and experimental analysis of temperature distribution and melt flow in fiber laser welding of inconel 625, Int. J. Advanced Manufacturing and Technology 121 (2022) 765. https://doi.org/10.21203/rs.3.rs-982749/v1

I. Tlili, E. M. Barhoumi, P. C. Okonkwo, I. B. Belgacem & M. Zghaibeh, “Optimal sizing of photovoltaic systems based green hydrogen refueling stations case study Oman”, Int. J. Hydrogen Energy. 47 (2022) 31964. https://doi.org/10.1016/j.ijhydene.2022.07.140

H. A. Hejazi, M. I. Khan, A. Raza, K. Smida & S. U. Khan, I. Tlili, “Inclined surface slip flow of nanoparticles with subject to mixed convection phenomenon: fractional calculus applications”, J. Indian Chemical Society. 99 (2022) 100564. https://doi.org/10.1016/j.jics.2022.100564

A. Moutsoglou & T. S. Chen, “Buoyancy effects in boundary layers on inclined continuous moving sheets”, J. Heat Transfer. 102 (1980) 371. https://doi.org/10.1115/1.3244292

K. Vajravelu, “Convection heat transfer at a stretching sheet with suction or blowing”, Journal of Mathematical Analysis and Applications 188 (1994) 1002. https://doi.org/10.1006/jmaa.1994.1476

S. Das, R. N. Jana & O. D. Makinde, “Magneto hydrodynamic mixed convective slip flow over an inclined porous plate with viscous dissipation and joule heating”, Alex. Eng. J. 54 (2015) 251. https://doi.org/10.1016/j.aej.2015.03.003

M. B. Ashraf, T. Hayat, A. Alsadi & S. A. Shehzad, “Convective heat and mass transfer in MHD mixed convection of flow of Jeffery nanofluid over a radially stretching surface with thermal radiation”, J. Cent. South. Univ. 22 (2015) 1114. http://dx.doi.org/10.1007/s11771-015-2623-6

M. B. Ashraf, T. Hayat & A. Alsadi, “Mixed convection flow of Casson fluid over a stretching sheet with convective boundary conditions and hall effect”, Boundary Value Problems 2017 (2017)137. https://doi.org/10.1186/s13661-017-0869-7

T. Hayat & S. Nadeem, “The effects of MHD and buoyancy on Hematite water-based fluid past a convectively heated stretching sheet”, Neural Comput. & Applic. 31 (2019) 1083. https://doi.org/10.1007/s00521-017-3139-9

D. Habib, N. Salmat, S. Hussain, B. Ali & S. Abdal, “Significance of Stephen blowing and Lorentz force on dynamics of Prandtl nanofluid via Keller box approach”, Int. J. Communications in Heat and Mass transfer 128 (2021) 105599. https://doi.org/10.1016/j.icheatmasstransfer.2021.105599

F. O. Akinpelu, R. A. Oderinu & A. D. Ohaegbue, “Analysis of hydromagnetic double exothermic chemical reactive flow with convective cooling through a porous medium under bimolecular kinetics”, J. Nig. Soc. Phys. Sci. 4 (2022) 525. https://doi.org/10.46481/jnsps.2022.525

M. Asif, S. U. Haq, S. Islam, T. A. Alkanhal, Z. A. Khan, I. Khan &K. S. Nisar “Unsteady flow of fractional fluid between two parallel walls with arbitrary wall shear stress using Caputo–Fabrizio derivative”, Symmetry 11 (2019) 449. https://doi.org/10.3390/sym11040449

L. A. Lund, Z. Omar, S. O. Alharbi, I. Khan & K. S. Nisar, “Numerical investigation of multiple solutions for Caputo fractional-order-two dimensional magnetohydrodynamic unsteady flow of generalized viscous fluid over a shrinking sheet using the adams-type predictor-corrector method”, Coatings 9 (2019) 548. https://doi.org/10.3390/coatings9090548

S. Liao, “An optimal Homotopy – analysis approach for strongly nonlinear equations”, Commu. Nonlinear Sci. Num. Simu. 15 (2009) 2003. http://dx.doi.org/10.1016/j.cnsns.2009.09.002

T. Fan & X. You, “Optimal homotopy analysis method for nonlinear differential equations in the boundary layer”, Numer. Algor. 62 (2013) 337. https://doi.org/10.1007/s11075-012-9587-5

R. A. Van Gorder“ Optimal homotopy analysis and control of error for implicitly defined fully nonlinear differential equations”, Numer. Algor. 81 (2019) 181. https://doi.org/10.1007/s11075-018-0540-0

M. Shoaib, G. Zubair, M. A. Z. Raja, K. S. Nisar, A.-H Abdel-Aty & I. S. Yahia, “Study of 3-D Prandtl nanofluid flow over a convectively heated sheet: a stochastic intelligent technique”, Coatings 12 (2022) 24. https://doi.org/10.3390/coatings12010024

H. Vaidya, K. Prasad, M. I. Khan., F. Mebarek-Oudina, I. Tlili, C. Rajashekhar, S. Elattar., M. I. Khan & S. G. Al-Gamdi, “Combined effects of chemical reaction and variable thermal conductivity on MHD peristaltic flow of Phan-Thien-Tanner liquid through inclined channel”, Case Studies in Thermal Engineering 36 (2022) 102214. https://doi.org/10.1016/j.csite.2022.102214

S. Li, S. M. Sajadi, K. A. M. Alharbi, M. El-Shorbagy & I. Tlili, “The molecular dynamics study of vacancy defect influence on carbon nanotube performance as drug delivery system”, Engineering Analysis with Boundary Elements 143 (2022) 109. https://doi.org/10.1016/j.enganabound.2022.06.006

A. M. Alqahtani, S. M. Sajadi, A. S. Al-Johani, K. A. M. Alharbi, A. E. S. Ahmed & I. Tlili, “The atomic obstacle size influence on the Hydrogen flow inside a nanochannel: a molecular dynamics approach to predict the fluid atomic arrangements”, Engineering Analysis with Boundary Elements 143 (2022) 547. https://doi.org/10.1016/j.enganabound.2022.06.027

C. N. Guled, J. V. Tawade, M. M. Nandeppanavar & A. R. Saraf, “MHD slip flow and heat transfer of UCM fluid with the effect of suction/injection due to stretching sheet: OHAM solution”, Heat Transfer 51 (2022) 3201. https://doi.org/10.1002/htj.22444

V. Ramanjini, G. G. Krishna, S. R. Mishra, S. V. S. Kumari & H. K. Sree, “An unsteady axisymmetric Williamson nanofluid over a radially stretching Riga plate in the presence of mixed convection and thermal radiation”, Partial Differential Equations in Applied Mathematics 6 (2022) 100456. https://doi.org/10.1016/j.padiff.2022.100456

P. Mallikarjuna & K. B. Umadevi, “Impact of an MHD Cattaneo-Christov model for a Williamson fluid flow across a wedge with a convective boundary condition: Homotopy analysis method”, Int. J. Ambient Energy. 44 (2023) 1097. https://doi.org/10.1080/01430750.2022.2162964

Published

2023-10-08

How to Cite

Unsteady Prandtl nanofluidic flow through stretching sheet due to mixed convection, first-order slip, and chemical reaction. (2023). Journal of the Nigerian Society of Physical Sciences, 5(4), 1140. https://doi.org/10.46481/jnsps.2023.1140

Issue

Volume 5, Issue 4, November 2023

Section

Original Research
Copyright & Licensing

Copyright (c) 2023 V. Ramanjini, G. Gopi Krishna, Mani Ramanuja, Hari Kamala Sree, S. R. Mishra

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Unsteady Prandtl nanofluidic flow through stretching sheet due to mixed convection, first-order slip, and chemical reaction. (2023). Journal of the Nigerian Society of Physical Sciences, 5(4), 1140. https://doi.org/10.46481/jnsps.2023.1140