On the multiplicity order of spinnable star-like transformation semigroup Tw*n

Authors

  • Sulaiman Awwal AKINWUNMI Department of Mathematics and Statistics, Federal University of Kashere, Gombe, Nigeria
  • Garba Risqot IBRAHIM Department of Mathematics and Statistics, Kwara State University Malete, Kwara, Nigeria
  • Adenike Olusola ADENIJI Department of Mathematics, University of Abuja, Abuja, Nigeria
  • David Opeoluwa OYEWOLA Department of Mathematics and Statistics, Federal University of Kashere, Gombe, Nigeria

Keywords:

Transformation semi-group, Multiplicity order, Geometry, Star-like spinnable

Abstract

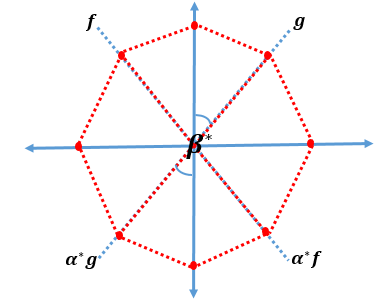
The application of graph theory has gained significant traction within the realm of the algebraic theory of semigroups. This study delves into exploring the geometric properties of the star-like transformation semigroup \alpha\omega_n^*, a distinctive category of transformation, and delineates a tropical graph (curve) by elucidating its algebraic and tropical structure. Through this investigation, various tropical properties are established, offering insights into the graph theory aspects of star-like spinnable T\omega_n^* transformation semigroups. Consequently, the objective of this research is to delineate and characterize several tropical and combinatorial functions applicable to T\omega_n^*.

Dimensions

O. Ganyushkin & V. Mazorchuk, Classical finite transformation semigroups; An Introduction, 2009th edition, Springer, London, 2009. https://doi.org/10.1007/978-1-84800-281-4.

J. M. Howie Fundamentals of semigroup theory , 1st Edition, London Mathematical Society Monographs, 12, 1996. ISBN-13: 9780198511946

R. Kehinde & A. Umar “On the semigroup of partial isometries of finite chain contraction mapping”, Australian journal 44 (2010) 184. https://doi.org/10.1080/00927872.2014.984838.

A. Umar, “Some combinatorial in the theory of partial transformation semigroups”, Algebra and Discrete Mathematics 2 17 (2014) 110. https://www.researchgate.net/publication/287232365.

S. A. Akinwunmi, M. M. Mogbonju & G. R. Ibrahim,“Some characterizations of equivalence relation on contraction mappings”, Scientific African 10 (2020) e00643. https://doi.org/10.1016/j.sciaf.2020.e00643.

A. Laradji & A. Umar, “On certain finite semigroups of order-decreasing transformations I”, Semigroup Forum, Springer 69 (2004) 184. http://dx.doi.org/10.1007/s00233-004-0101-9.

D. Maclagan, Tropical geometry, Lecture notes series, (2017).

G. Mikhalkin, “Tropical geometry and its applications”, Lecture note series, University of Texas 1 (2006). https://doi.org/10.48550/arXiv.math/0601041.

E. Brugalle & K. Shaw, “A bit of tropical geometry”, The American Mathematical monthly 121 (2014) 563. https://doi.org/10.48550/arXiv.1311.2360.

E. Brugalle, I. Itenberg, G. Mikhalkin & K. Shaw,Brief introduction to tropical geometry, Proceedings of 21st Gokova Geometry-topology conference, 2014. https://doi.org/10.48550/arXiv.1502.05950.

I. Protrka An invitation to combinatorial tropical geometry, 2017. https://doi.org/10.5592/CO%2FCCD.2016.05.

G. Mikhalkin “Enumerative tropical algebraic geometry in R2”, J. Amer. Math. Soc. 18 (2004) 313. https://doi.org/10.48550/arXiv.math/0312530.

S. Imre, Recognizable set with multiplicities in the tropical semiring, mathematical foundations of computer science, Springer, Berlin Heidelberg, 1988. https://doi.org/10.1007/BFb0017135.

Star-like spinnable model.

Published

2024-03-06

How to Cite

On the multiplicity order of spinnable star-like transformation semigroup Tw*n. (2024). Journal of the Nigerian Society of Physical Sciences, 6(1), 1962. https://doi.org/10.46481/jnsps.2024.1692

Issue

Volume 6, Issue 1, February 2024

Section

Mathematics & Statistics
Copyright & Licensing

Copyright (c) 2023 Sulaiman Awwal AKINWUNMI, Garba Risqot IBRAHIM, Adenike Olusola ADENIJI, David Opeoluwa OYEWOLA

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

On the multiplicity order of spinnable star-like transformation semigroup Tw*n. (2024). Journal of the Nigerian Society of Physical Sciences, 6(1), 1962. https://doi.org/10.46481/jnsps.2024.1692