Transmuted cosine Topp-Leone G family of distributions: properties and applications
Keywords:
Tope-Leone G, Cosine-G, Transmuted-G, Monte-Carlo simulation, Maximum likelihood estimationAbstract
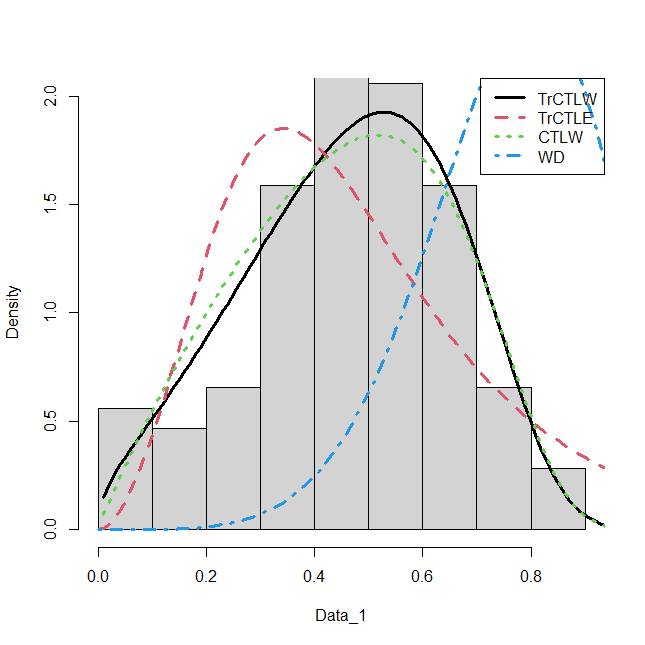
In contemporary data analysis, the need for flexible and adaptable probabilistic models capable of capturing complex dependencies and tail behaviors in real-life datasets has become increasingly apparent. In light of this demand, A new trigonometric generalized family of distribution, the "Transmuted Cosine Topp-Leone G Family" is proposed in this study. Established on the foundations of, this family combines the adaptability of the Topp-Leone distribution with the periodicity of the cosine function and transmutation theory to produce a flexible framework that may be used to represent a wide range of real-life phenomena. We derive some of the statistical properties of the introduced family such as; survival and hazard functions, and moment and moment-generating functions. Moreover, the model parameters are estimated using the Maximum Likelihood method, and a Monte Carlo simulation was performed to ascertain the behavior and the consistency of the estimates. We investigate the shape of the distribution parameters which results in distributions with left-skew, right-skew, increasing, and decreasing shapes. Lastly, through empirical demonstrations, we showcase the efficacy of the family TrCTLG models in fitting lifetime datasets.
Published
How to Cite
Issue
Section
Copyright (c) 2024 Abdulhameed Ado Osi, Sani Ibrahim Doguwa, Abubakar Yahaya, Yahaya Zakari, Abubakar Usman

This work is licensed under a Creative Commons Attribution 4.0 International License.






