Mathematical modelling of concentration profiles for species transport through the single and the interconnected multiple-compartment systems

Authors

  • Oludare Adedire University of Jos
  • J. N. Ndam Department of Mathematics, University of Jos, Nigeria

Abstract

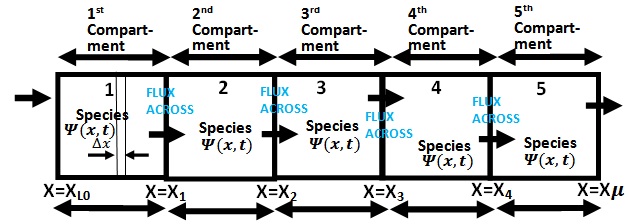
In this research work, we investigate the concentration profiles in the single and the interconnected multiple-compartment systems with sieve partitions for the transport of chemical species with second order chemical reaction kinetics. With assumption of unidirectional transport of chemical species and constant physical properties with same equilibrium contant, the developed partial differential equations representing the two systems are spatially discretized using the Method of Lines (MOL) technique and the resulting semi-discrete system of ODEs are solved using MATLAB ode15s solver. The results show that the interconnected multiple-compartment system has lower concentration profile than the single-compartment system for different values of diffusivities.

Dimensions

Adedire, O., Ndam, J. N. (2019). Mathematical modeling of chlorine decay through water and intermediate pseudomonas aeruginosa in multiple-compartment isothermal reactor. Journal of Advanced Mathematical Models and Applications, 4(2), 167-178.

Chepurniy, N., Savage, S. B.(1975). Effect of diffusion on concentration profiles in a solar pond Journal of solar energy, 17, 203-205, doi: 10.1016/0038-092x(75)90061-4

Critelli, R.A.J., Bertotti, M., Torresi, R. M. (2018). Probe effects on concentration profiles in the diffusion layer: Computational modeling and near-surface pH measurements using microelec- trodes. Journal of electrochemical acta, 511-521, doi: 10.1016/j.electacta.2018.09.157

Gǎiseanu, F. (1983). On the diffusion profile of boron in silicon at high concentrations. Journal of physical state solid, 77, 51-61, doi: 10.1002/pssa.2210770164

Kreiss,H.,andLorenz,J.(1989).Initial-boundary valueproblems and the Navier-StokesEquation. San Diego: Academic Press, Inc.

Lasaga,A.C.(1981).Influenceofdiffusioncouplingondiageneticconcentrationprofiles.American Journal of Science, 281, 553-575.

Leute, A.C. (2010). The influence of the ideal mixing entropy on concentration profiles and diffusion paths in ternary systems. American Journal of Science, 281, 553-575, doi: 10.1016/j.jpcs.2010.08.018

Michl,B.,Schön,J.,Warta,W.,Schubert,M.C.(2013).Theimpactofdifferentdiffusiontemper- ature profiles on iron concentrations and carrier lifetimes in multicrystalline silicon wafers.IEEE Journal of photovoltaics, 71(12), 1768-1773, doi: 10.1109/jphotov.2012.2231726

Peev, G., Rouseva.M.(1994). A model for the concentration profile of PxOy in the interwafer gas phase on phosphorus doping of silicon using a solid planar diffusion source. Journal of modelling simulation material Science Engineering, 2, 1143-1152, doi: 10.1088/0965-0393/2/6/006

Rothhaar, U., and Oechsner, H. (1995). Determination of diffusion induced concentration profiles in Cr203 films on ceramic Al2Oa by Auger sputter depth profiling.FreseniusJournalofAnalytical Chemistry, 353, 533-535, doi: 10.1007/BF00321316

Schulz, R., Yamamoto, K., Klossek, A., Rancan, F., Vogt, A., Schütte, C., Rühl, E.,and Netz, R. R.(2019). Modeling of Drug Diffusion Based on Concentration Profiles in Healthy and Damaged Human Skin. Biophysical Journal, 117(5), 998-1008, doi: 10.1016/j.bpj. 2019.07.027

Strikwerda, J. (1977). Initial boundary value problems for incomplete parabolic systems. Journal of Communications on Pure and Applied Mathematics, 30, 797-822.

Tyrrell, H.J.V. (1964). The origin and present status of Fick’s diffusion law. Journal of Chemical Education, 41(7), 397-400.

Wang, W., andLo. Y. (1983). Profile estimation of high concentration Arsenic or Boron diffusion in Silicon. Journal of IEEE Transactions on Electron Devices, 30(12), 1828-1831, doi: 10.1109/t- ed.1983.21453.

Yang, T.H., Lue, S. J.(2013). Coupled concentration-dependent diffusivities of ethanol/water mix- tures through a polymeric membrane: Effect on pervaporative flux and diffusivity profiles. Journal of Membrane Science, 443, 1-9, doi: 10.1016/j.memsci.2013.05.002.

Published

2020-05-14

How to Cite

Mathematical modelling of concentration profiles for species transport through the single and the interconnected multiple-compartment systems . (2020). Journal of the Nigerian Society of Physical Sciences, 2(2), 61-68. https://doi.org/10.46481/jnsps.2020.72

Issue

Volume 2, Issue 2, May 2020

Section

Original Research
Copyright & Licensing

How to Cite

Mathematical modelling of concentration profiles for species transport through the single and the interconnected multiple-compartment systems . (2020). Journal of the Nigerian Society of Physical Sciences, 2(2), 61-68. https://doi.org/10.46481/jnsps.2020.72