Optimizing discrete dutch auctions with time considerations: a strategic approach for lognormal valuation distributions

Raja Aqib Shamim; Majid Khan Majahar Ali

doi:10.46481/jnsps.2025.2291

Authors

Raja Aqib Shamim School of Mathematical Sciences, Universiti Sains Malaysia, 11800, Pulau Penang, Malaysia | Department of Mathematics, University of Kotli, 11100, Azad Jammu and Kashmir, Pakistan https://orcid.org/0000-0002-2531-1249
Majid Khan Majahar Ali
[email protected]
School of Mathematical Sciences, Universiti Sains Malaysia, 11800, Pulau Penang, Malaysia https://orcid.org/0000-0002-5558-5929

Keywords:

Auctions, Lognormal distribution, Nonlinear programming, Discrete Dutch auction, Revenue optimization

Abstract

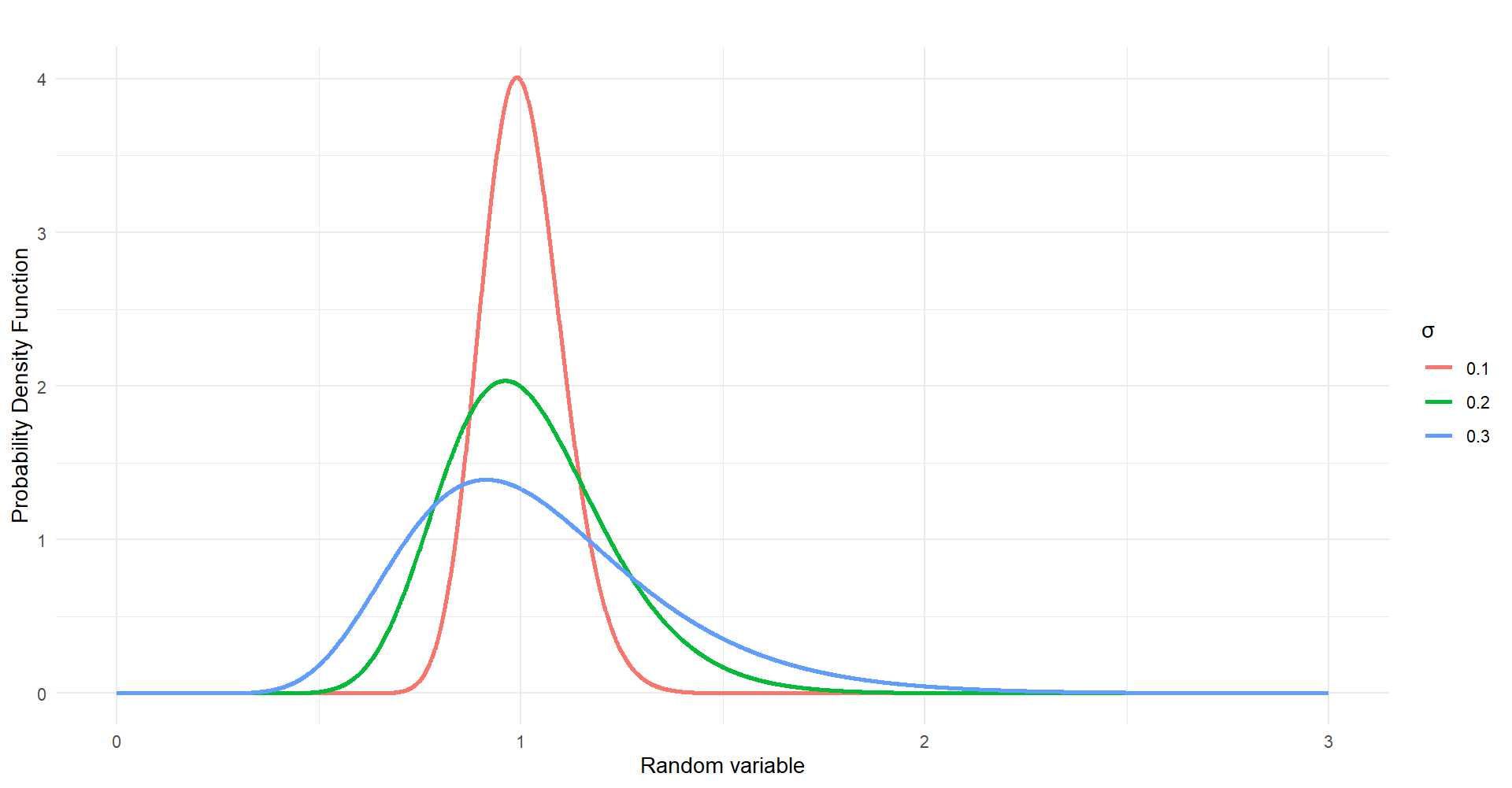
This research deviates from usual studies in auction literature primarily focused on maximizing expected revenue. Instead, we concentrate on the strategic design of discrete Dutch auctions in the context of bidder emotional attachment, wherein valuations follow a lognormal distribution. Our objective is to attain an optimal balance between the auctioned object’s selling price and the auction duration, ultimately maximizing the auctioneer’s expected revenue per unit of time. Our proposed models exhibit significantly higher average revenues per unit of time than counterparts neglecting time considerations and emotional attachment of the bidders. This achievement results from strategically reducing auction durations, enabling more auctions within the allotted time. This intentional trade-off ensures the marginal revenue decrease in shorter auctions is surpassed by the substantial increase in overall revenues from heightened auction frequency. Numerical results emphasize the utility of our modified discrete Dutch auction design, particularly in scenarios with a large number of bidders. Furthermore, increasing skewness in valuation distributions correlates with higher revenue per unit of time. Complete knowledge of the number of participating bidders is crucial, leading to a noticeable elevation in the auctioneer’s expected revenue per unit of time. However, the predictability of auction outcomes may be challenging, underscoring the nuanced nature of auction dynamics.

Dimensions

REFERENCES

[1] A. Cheema, D. Chakravarti & A. R. Sinha, “Bidding behavior in descending and ascending auctions”, Marketing Science 31 (2012) 779. https://doi.org/10.1287/mksc.1120.0730.

[2] M. T. Adam, A. Eidels, E. Lux & T. Teubner, “Bidding behavior in Dutch auctions: Insights from a structured literature review”, International Journal of Electronic Commerce 21 (2017) 363. https://doi.org/10.1080/10864415.2016.1319222.

[3] Z. Li, J. Yue & C. C. Kuo “Design of discrete dutch auctions with consideration of time”, European Journal of Operational Research 265 (2018) 1159. https://doi.org/10.1016/j.ejor.2017.08.043.

[4] P. Miettinen, “Information acquisition during a dutch auction”, Journal of Economic Theory 148 (2013) 1213. https://doi.org/10.1016/j.jet.2012.09.018.

[5] R. P. McAfee & J. McMillan, “Auctions with a stochastic number of bidders”, Journal of economic theory 43 (1987) 1 https://doi.org/10.1016/0022-0531(87)90113-X.

[6] M. A. Robinson & R. Robinson, “Dutch-auction IPOs:institutional development and underpricing performance”, Journal of Economics and Finance 36 (2012) 521. https://doi.org/10.1007/s12197-010-9166-3.

[7] V. Gretschko, A. Rasch & A. Wambach, “On the strictly descending multi-unit auction”, Journal of Mathematical Economics 50 (2014) 79. https://doi.org/10.1016/j.jmateco.2013.10.009.

[8] C. Brown & K. Davis, “Taxes, tenders and the design of australian offmarket share repurchases”, Accounting & Finance 52 (2012) 109. https://doi.org/10.1111/j.1467-629X.2011.00445.x.

[9] M. Bichler, V. Grimm, S. Kretschmer & P. Sutterer, “Market design for renewable energy auctions: An analysis of alternative auction formats”, Energy Economics 92 (2020) 104904. https://doi.org/10.1016/j.eneco.2020.104904.

[10] G. Rubio-Domingo & P. Linares, “The future investment costs of offshore wind: An estimation based on auction results”, Renewable and Sustainable Energy Reviews 148 (2021) 111324. https://doi.org/10.1016/j.rser.2021.111324.

[11] H. Qiu, K. Zhu, N. C. Luong, C. Yi, D. Niyato & D. I. Kim, “Applications of auction and mechanism design in edge computing: A survey”, IEEE Transactions on Cognitive Communications and Networking 8 (2022) 1034. https://doi.org/10.1109/TCCN.2022.3147196.

[12] Z. Shi, C. de Laat, P. Grosso & Z. Zhao, “Integration of blockchain and auction models: A survey, some applications, and challenges”, IEEE Communications Surveys & Tutorials 25 (2022) 497. https://doi.org/10.1109/COMST.2022.3222403.

[13] H. Qiu & T. Li, “Auction method to prevent bid-rigging strategies in mobile blockchain edge computing resource allocation”, Future Generation Computer Systems, 128 (2022) 1. https://doi.org/10.1016/j.future.2021.09.031.

[14] N. Sharghivand, F. Derakhshan & N. Siasi, “A comprehensive survey on auction mechanism design for cloud/edge resource management and pricing”, IEEE Access 9 (2021) 126502. https://doi.org/10.1109/ACCESS.2021.3110914.

[15] Z. Li & C. C. Kuo, “Revenue-maximizing dutch auctions with discrete bid levels”, European Journal of Operational Research 215 (2011) 721. https://doi.org/10.1016/j.ejor.2011.05.039.

[16] Z. Li and C. C. Kuo, “Design of discrete dutch auctions with an uncertain number of bidders”, Annals of Operations Research 211 (2013) 255. https://doi.org/10.1007/s10479-013-1331-6.

[17] M. H. Rothkopf & R. M. Harstad, “On the role of discrete bid levels in oral auctions”, European Journal of Operational Research 74 (1994) 572. https://doi.org/10.1016/0377-2217(94)90232-1.

[18] M. Truong, A. Gupta, W. Ketter & E. V. Heck, “Buyers’ strategic behavior in b2b multichannel auction markets: When an online posted price channel is incorporated into a dutch auction system”, Information Systems Research 33 (2022) 1344. https://doi.org/10.1287/isre.2022.1180.

[19] A. Kambil & E. Van Heck, “Reengineering the dutch flower auctions:A framework for analyzing exchange organizations”, Information Systems Research, 9 (1998) 1. https://doi.org/10.1287/isre.9.1.1.

[20] M. Gallegati, G. Giulioni, A. Kirman & A. Palestrini, “What’s that got to do with the price of fish? buyers behavior on the ancona fish market”, Journal of Economic Behavior & Organization, 80 (2011) 20. https://doi.org/10.1016/j.jebo.2011.01.011.

[21] J. Yu, Discrete approximation of continuous allocation mechanisms, Ph.D. dissertation, Deaprtment of Humanities and Social Sciences, California Institute of Technology, California, USA, 1999. https://doi.org/10.7907/8SF1-5M95.

[22] W. H. Yuen, C. W. Sung & W. S. Wong, “Optimal price decremental strategy for dutch auctions”, Communications in Information and Systems 2 (2022) 411. https://doi.org/10.4310/CIS.2002.v2.n4.a6.

[23] R. Cassady, Auctions and auctioneering, University of California Press, California, USA, 1967, pp. 92-94. https://doi.org/10.1525/9780520322257.

[24] T. Nedelec, C. Calauzenes, V. Perchet & N. El Karoui, “Revenuemaximizing auctions: a bidder’s standpoint”, Operations Research, 70 (2022) 2767. https://doi.org/10.1287/opre.2022.2316.

[25] T. Chipty, S. R. Cosslett & L. F. Dunn, “A race against the clock: Auctioneer strategies and selling mechanisms in live outcry auctions”, Available at SSRN 2566616 1 (2015) 1. https://doi.org/10.2139/ssrn.2566616.

[26] M. T. Adam, G. Ku & E. Lux, “Auction fever: The unrecognized effects of incidental arousal”, Journal of Experimental Social Psychology, 80 (2019) 52. https://doi.org/10.1016/j.jesp.2018.07.009.

[27] M. T. Adam, J. Kramer, C. J¨ ahnig, S. Seifert, & C. Weinhardt, “Under-¨ standing auction fever: A framework for emotional bidding”, Electronic Markets, 21 (2011) 197. https://doi.org/10.1007/s12525-011-0068-9.

[28] M. T. P. Adam & J. Kramer, “Evaluating the emotional bidding frame-¨ work: new evidence from a decade of neurophysiology”, Electronic Markets, 32 (2022) 1529. https://doi.org/10.1007/s12525-022-00555-x.

[29] G. Ku, D. Malhotra & J. K. Murnighan, “Towards a competitive arousal model of decision-making: A study of auction fever in live and internet auctions”, Organizational Behavior and Human decision processes, 96 (2005) 89. https://doi.org/10.1016/j.obhdp.2004.10.001.

[30] M. Isaac, S. Pevnitskaya & K. S. Schnier, “Individual behavior and bidding heterogeneity in sealed bid auctions where the number of bidders is unknown”, Economic Inquiry, 50 (2012) 516. https://doi.org/10.1111/j.1465-7295.2011.00393.x.

[31] D. Levin & E. Ozdenoren, “Auctions with uncertain numbers of bidders”, Journal of Economic Theory, 118 (2004) 229. https://doi.org/10.1016/j.jet.2003.11.007.

[32] W. L. Winston, M. Venkataramanan & J. B. Goldberg, Introduction to mathematical programming, Duxbury Press, Belmont, CA, 2004. https://dl.acm.org/doi/abs/10.5555/1202147.

[33] E. Katok & A. M. Kwasnica, “Time is money: The effect of clock speed on seller’s revenue in dutch auctions”, Experimental Economics 11 (2008) 344. https://doi.org/10.1007/s10683-007-9169-x.

[34] E. Guerci, A. Kirman & S. Moulet, “Learning to bid in sequential dutch auctions”, Journal of Economic Dynamics and Control 48 (2014) 374. https://doi.org/10.1016/j.jedc.2014.09.029.

[35] E. David, A. Rogers, N. R. Jennings, J. Schiff, S. Kraus & M. H. Rothkopf, “Optimal design of english auctions with discrete bid levels”, ACM Transactions on Internet Technology 7 (2007) 12. https://doi.org/10.1145/1239971.1239976.

[36] J. Gallien & S. Gupta, “Temporary and permanent buyout prices in online auctions”, Management Science, 53 (2007) 814. https://doi.org/10.1287/mnsc.1060.0650.

[37] M. Gentry, T. Li & J. Lu, “Existence of monotone equilibrium in first price auctions with private risk aversion and private initial wealth”, Games and Economic Behavior 94 (2015) 214. https://doi.org/10.1016/ j.geb.2015.07.003.

[38] U. Bergmann & A. Konovalov, “Auction design and order of sale with budget-constrained bidders”, Experimental Economics 27 (2024) 36. https://doi.org/10.1007/s10683-023-09812-y.